Advertisements
Advertisements
प्रश्न
Solve the following question using appropriate Euclid’s axiom:
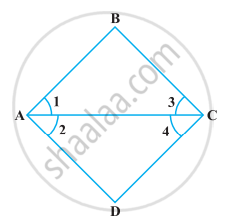
In the following figure, we have ∠ABC = ∠ACB, ∠3 = ∠4. Show that ∠1 = ∠2.

उत्तर
Given, ∠ABC = ∠ACB ...(i)
And ∠4 = ∠3 ...(ii)
According to Eulid’s axiom, if equals are subtracted from equals, then remainders are also equal.
On subtracting equation (ii) from equation (i), we get
∠ABC – ∠4 = ∠ACB – ∠3
⇒ ∠1 = ∠2
Now, in ABDC, ∠1 = ∠2
⇒ DC = BD ...[Sides opposite to equal angles are equal]
BD = DC.
APPEARS IN
संबंधित प्रश्न
Give a definition of the following term. Are there other terms that need to be defined first? What are they, and how might you define them?
line segment
How many planes can be made to pass through a line and a point not on the line?
The three steps from solids to points are ______.
A pyramid is a solid figure, the base of which is ______.
Euclid stated that all right angles are equal to each other in the form of ______.
The boundaries of the solids are curves.
The things which are double of the same thing are equal to one another.
“For every line l and for every point P not lying on a given line l, there exists a unique line m passing through P and parallel to l ” is known as Playfair’s axiom.
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have ∠1 = ∠2, ∠2 = ∠3. Show that ∠1 = ∠3.
Solve the following question using appropriate Euclid’s axiom:
In the following figure, if OX = `1/2` XY, PX = `1/2` XZ and OX = PX, show that XY = XZ.