Advertisements
Advertisements
प्रश्न
Suppose an attractive nuclear force acts between two protons which may be written as F=Ce−kr/r2. Write down the dimensional formulae and appropriate SI units of C and k.
उत्तर
Given, nuclear force of attraction,
\[F = C\frac{e^{- Kr}}{r^2}\]
Here e−Kr is just a pure number, i.e. a dimensionless quantity. So,
\[\left[ C \right] = \left[ F \right] \times \left[ r^2 \right]\]
\[\left[ C \right] = \left[ {MLT}^{- 2} \right] \times \left[ L^2 \right]\]
\[\left[ C \right] = \left[ {ML}^3 T^{- 2} \right]\]
C = kg ⋅ m3 ⋅ s −2
\[\text{ And } \left[ K \right] = \frac{1}{\left[ r \right]} = \left[ L^{- 1} \right]\]
SI units: m−1
APPEARS IN
संबंधित प्रश्न
Check that the ratio ke2/G memp is dimensionless. Look up a Table of Physical Constants and determine the value of this ratio. What does the ratio signify?
Suppose that the particle is an electron projected with velocity vx = 2.0 × 106 m s−1. If E between the plates separated by 0.5 cm is 9.1 × 102 N/C, where will the electron strike the upper plate? (|e| = 1.6 × 10−19 C, me = 9.1 × 10−31 kg)
Two equal charges are placed at a separation of 1.0 m. What should be the magnitude of the charges, so that the force between them equals the weight of a 50 kg person?
Find the electric force between two protons separated by a distance of 1 fermi (1 fermi = 10−15 m). The protons in a nucleus remain at a separation of this order.
Two charges 2.0 × 10−6 C and 1.0 × 10−6 C are placed at a separation of 10 cm. Where should a third charge be placed, such that it experiences no net force due to these charges?
Consider a gold nucleus to be a sphere of radius 6.9 fermi in which protons and neutrons are distributed. Find the force of repulsion between two protons situated at largest separation. Why do these protons not fly apart under this repulsion?
Two identical balls, each with a charge of 2.00 × 10−7 C and a mass of 100 g, are suspended from a common point by two insulating strings, each 50 cm long. The balls are held at a separation 5.0 cm apart and then released. Find.
(a) the electric force on one of the charged balls
(b) the components of the resultant force on it along and perpendicular to the string
(c) the tension in the string
(d) the acceleration of one of the balls. Answers are to be obtained only for the instant just after the release.
Two small spheres, each with a mass of 20 g, are suspended from a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the separation between the balls at equilibrium is found to be 4 cm. Find the charge on each sphere.
A particle with a charge of 2.0 × 10−4 C is placed directly below and at a separation of 10 cm from the bob of a simple pendulum at rest. The mass of the bob is 100 g. What charge should the bob be given so that the string becomes loose?
Repeat the previous problem if the particle C is displaced through a distance x along the line AB.
Two particles A and B possessing charges of +2.00 × 10−6 C and of −4.00 × 10−6 C, respectively, are held fixed at a separation of 20.0 cm. Locate the points (s) on the line AB, where (a) the electric field is zero (b) the electric potential is zero.
How much work has to be done in assembling three charged particles at the vertices of an equilateral triangle, as shown in the figure?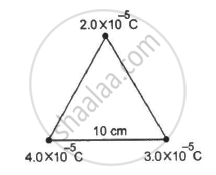
Three charges +Q, q, +Q are placed respectively, at distance, 0, d/2 and d from the origin, on the X-axis. If the net force experienced by +Q, placed at x = 0, is zero then value of q is ____________.
The force between two charges 0.06 m apart is 5 N. If each charge is moved towards the other by 0.01 m, then the force between them will become ____________.
SI unit of permittivity of free space is ______.
Two charges of equal magnitudes kept at a distance r exert a force F on each other. If the charges are halved and distance between them is doubled, then the new force acting on each charge is ______.
Coulomb's law is given by F = k q1q2 rn where n is
Which of the following statements about nuclear forces is not true?
Two point charges +2 C and +6 C repel each other with a force of 12 N. If a charge of -4 C is given to each of these charges, then the force now is ______.
