Advertisements
Advertisements
प्रश्न
The angles A, B, C and D of a quadrilateral are in the ratio 2 : 3 : 2 : 3. Show this quadrilateral is a parallelogram.
उत्तर १
Given, Angles of a quadrilateral are in the ratio 2 : 3 : 2 : 3
i.e. A : B : C : D are in the ratio
2 : 3 : 2 : 3
To prove: Quadrilateral ABCD is a parallelogram
Proof: Let us take ∠A = 2x, ∠B = 3x, ∠C = 2x and ∠D = 3x
We know, that the sum of interior angles of a quadrilateral = 360°
⇒ ∠A + ∠B + ∠C + ∠D = 360°
⇒ 2x + 3x + 2x + 3x = 360°
⇒ 10x = 360°
⇒ x = `360^circ/10 = 36^circ`
∴ ∠A = ∠C = 2x = 2 × 360° = 72°
∠B = ∠D = 3x = 3 × 36° = 360°
Now, A quadrilateral ABCD is considered as a parallelogram.
(i) When opposite angles are equal,
i.e. ∠A = ∠C = 72° and ∠B = ∠D = 108°
(ii) When adjacent angles are supplementary
i.e. ∠A + ∠B = 180°
and ∠C = ∠D = 180°
⇒ 72° + 108° and 72° + 108° = 180°
⇒ 180° = 180° and 180° = 180°
Since quadrilateral ABCD fulfills the conditions.
∴ Quadrilateral ABCD is a parallelogram.
उत्तर २
The sum of all angles in a quadrilateral is 360∘. Let the angles of the quadrilateral be 2x, 3x, 2x, and 3x.
Using the sum of the angles:
2x + 3x + 2x + 3x = 360∘.
Simplify: 10x = 360∘
x = 36∘
Substitute x = 36∘ into the expressions for the angles:
- 2x = 2 × 36 = 72∘
- 3x = 3 × 36 = 108∘
Thus, the angles are: 72∘, 108∘, 72∘, 108∘
In a parallelogram, opposite angles are equal. Here:
- One pair of opposite angles: 72∘, 72∘,
- Another pair of opposite angles: 108∘, 108∘
Since opposite angles are equal, the quadrilateral satisfies the property of a parallelogram.
The given quadrilateral is a parallelogram because its opposite angles are equal
APPEARS IN
संबंधित प्रश्न
Define the following term Convex Quadrilateral .
In a quadrilateral, define of the following Opposite sides .
Three angles of a quadrilateral are equal. Fourth angle is of measure 150°. What is the measure of equal angles.
The four angles of a quadrilateral are as 3 : 5 : 7 : 9. Find the angles.
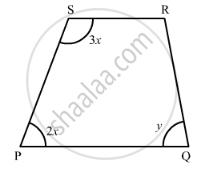
In the given figure, PQRS is an isosceles trapezium. Find x and y.
The consecutive sides of a quadrilateral have
Which of the following quadrilateral is not a rhombus?
Use the information given in the following figure to find the value of x.

The angles A, B, C and D of a trapezium ABCD are in the ratio 3: 4: 5: 6. Le. ∠A : ∠B : ∠C : ∠D = 3:4: 5 : 6. Find all the angles of the trapezium. Also, name the two sides of this trapezium which are parallel to each other. Give reason for your answer.
Two adjacent angles of a parallelogram are 70° and 110° respectively. Find the other two angles of it.
Write two conditions that will make the adjoining figure a square.
If APB and CQD are two parallel lines, then the bisectors of the angles APQ, BPQ, CQP and PQD form ______.
Which of the following is not true for a parallelogram?
In quadrilateral WXYZ, the pairs of opposite angles are ______.
Number of angles less than 180° in figure is ______ and their names are ______.
The common part between the two angles BAC and DAB in figure is ______.
Using the information given, name the right angles in part of figure:
RT ⊥ ST
Using the information given, name the right angles in part of figure:
OP ⊥ AB
Can we have two obtuse angles whose sum is a complete angle? Why or why not?
Draw a rough sketch of a quadrilateral PQRS. Draw its diagonals. Name them. Is the meeting point of the diagonals in the interior or exterior of the quadrilateral?