Advertisements
Advertisements
प्रश्न
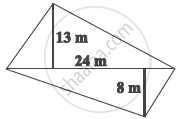
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
उत्तर
It is given that,
Length of the diagonal, d = 24 m
The lengths of the perpendiculars, h1 and h2, from the opposite vertices to the diagonal are h1 = 8 m and h2 = 13 m
Area of the quadrilateral = `1/2` d(h1 + h2)
= `1/2` (24m) × (13m + 8cm)
= `1/2` (24 m) (21 m)
= 252 m2
Thus, the area of the field is 252 m2
APPEARS IN
संबंधित प्रश्न
The diagonal of a rectangular plot is 34 m and its perimeter is 92 m. Find its area.
How many tiles, each of area 400 cm2, will be needed to pave a footpath which is 2 m wide and surrounds a grass plot 25 m long and 13 m wide?
Find the area and the perimeter of a square with diagonal 24 cm. [Take √2 = 1.41 ]
A quadrilateral field of unequal has a longer diagonal with 140m. The perpendiculars from opposite vertives upon this diagonal are 20m and 14m. Find the area of the field.
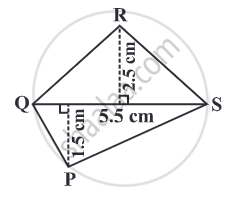
Find the area of quadrilateral PQRS.
Vertices of given triangles are taken in order and their areas are provided aside. Find the value of ‘p’.
| Vertices | Area (sq.units) |
| (p, p), (5, 6), (5, –2) | 32 |
In the following, find the value of ‘a’ for which the given points are collinear
(2, 3), (4, a) and (6, – 3)
Find the value of k, if the area of a quadrilateral is 28 sq. units, whose vertices are (– 4, – 2), (– 3, k), (3, – 2) and (2, 3)
When proving that a quadrilateral is a parallelogram by using slopes you must find
If the diagonal d of a quadrilateral is doubled and the heights h1 and h2 falling on d are halved, then the area of quadrilateral is ______.
