Advertisements
Advertisements
प्रश्न
The monthly expenditure on milk in 200 families of a Housing Society is given below:
| Monthly Expenditure (in ₹) |
1000 – 1500 | 1500 – 2000 | 2000 – 2500 | 2500 – 3000 | 3000 – 3500 | 3500 – 4000 | 4000 – 4500 | 4500 – 5000 |
| Number of families | 24 | 40 | 33 | x | 30 | 22 | 16 | 7 |
Find the value of x and also, find the median and mean expenditure on milk.
The monthly expenditure on milk in 200 families of a Housing Society is given below:
| Monthly Expenditure (in Rs.) | 1000 - 1500 | 1500 - 2000 | 2000 - 2500 | 2500 - 3000 | 3000 - 3500 | 3500 - 4000 | 4000 - 4500 | 4500 - 5000 |
| Number of families | 24 | 40 | 33 | x | 30 | 22 | 16 | 7 |
Find the value of x and also find the mean expenditure.
उत्तर
Given, Total number of families = 200
∴ 24 + 40 + 33 + x + 30 + 22 + 16 + 7 = 200
`\implies` x = 200 – 172 = 28
| Monthly expenditure (in ₹) (C.I.) |
Number of families (fi) |
Mid value (xi) |
xifi | C.F. |
| 1000 – 1500 | 24 | 1250 | 30,000 | 24 |
| 1500 – 2000 | 40 | 1750 | 70,000 | 64 |
| 2000 – 2500 | 33 | 2250 | 74,250 | 97 |
| 2500 – 3000 | 28 | 2750 | 77,000 | 125 |
| 3000 – 3500 | 30 | 3250 | 97,500 | 155 |
| 3500 – 4000 | 22 | 3750 | 82,500 | 177 |
| 4000 – 4500 | 16 | 4250 | 68,000 | 193 |
| 4500 – 5000 | 7 | 4750 | 33,250 | 200 |
| `bb(N = sumf_i = 200)` | `bb(sumx_i f_i = 5,32,500)` |
Mean = `(sumx_i f_i)/(sumf_i)`
= `(5,32,500)/200`
= ₹ 2662.5
Now, `N/2` = `200/2` = 100th
100th observation lies in class 2500 – 3000, which is known as median class.
l = lower limit of median class = 2500
N = number of families = 200
f = frequency of median class = 28
C.F. = cumulative frequency of the class preceding the median class = 97
h = class size = 500
Median = `l + ((N/2 - C.F.))/f xx h`
Median = `2500 + ((200/2 - 97))/28 xx 500`
= `2500 + (100 - 97)/28 xx 500`
= `2500 + 3/7 xx 125`
= 2500 + 53.571
= 2553.571
APPEARS IN
संबंधित प्रश्न
The lengths of 40 leaves of a plant are measured correct to the nearest millimeter, and the data obtained is represented in the following table:
| Length (in mm) | Number of leaves |
| 118 − 126 | 3 |
| 127 – 135 | 5 |
| 136 − 144 | 9 |
| 145 – 153 | 12 |
| 154 – 162 | 5 |
| 163 – 171 | 4 |
| 172 – 180 | 2 |
Find the median length of the leaves.
(Hint: The data needs to be converted to continuous classes for finding the median, since the formula assumes continuous classes. The classes then change to 117.5 − 126.5, 126.5 − 135.5… 171.5 − 180.5)
A life insurance agent found the following data for distribution of ages of 100 policy holders. Calculate the median age, if policies are given only to persons having age 18 years onwards but less than 60 years.
| Age (in years) | Number of policy holders |
| Below 20 | 2 |
| 20 - 25 | 4 |
| 25 - 30 | 18 |
| 30 - 35 | 21 |
| 35 - 40 | 33 |
| 40 - 45 | 11 |
| 45 - 50 | 3 |
| 50 - 55 | 6 |
| 55 - 60 | 2 |
If the median of the following data is 32.5, find the missing frequencies.
| Class interval: | 0 - 10 | 10 - 20 | 20 - 30 | 30 - 40 | 40 - 50 | 50 - 60 | 60 - 70 | Total |
| Frequency: | f1 | 5 | 9 | 12 | f2 | 3 | 2 | 40 |
Compute the median for the following data:
| Marks | No. of students |
| More than 150 | 0 |
| More than 140 | 12 |
| More than 130 | 27 |
| More than 120 | 60 |
| More than 110 | 105 |
| More than 100 | 124 |
| More than 90 | 141 |
| More than 80 | 150 |
A data has 25 observations arranged in a descending order. Which observation represents the median?
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
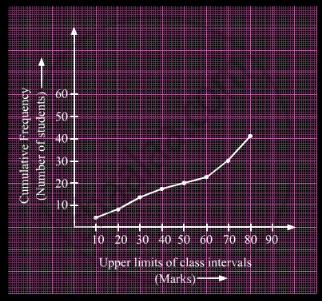
Calculate the median of the following distribution:
| No. of goals | 0 | 1 | 2 | 3 | 4 | 5 |
| No. of matches | 2 | 4 | 7 | 6 | 8 | 3 |
The median of the following data is 525. Find the values of x and y, if the total frequency is 100.
| Class interval | Frequency |
| 0 – 100 | 2 |
| 100 – 200 | 5 |
| 200 – 300 | x |
| 300 – 400 | 12 |
| 400 – 500 | 17 |
| 500 – 600 | 20 |
| 600 – 700 | y |
| 700 – 800 | 9 |
| 800 – 900 | 7 |
| 900 – 1000 | 4 |
Heights of 50 students of class X of a school are recorded and following data is obtained:
| Height (in cm) | 130 – 135 | 135 – 140 | 140 – 145 | 145 – 150 | 150 – 155 | 155 – 160 |
| Number of students | 4 | 11 | 12 | 7 | 10 | 6 |
Find the median height of the students.
The median of 100 observations grouped in classes of equal width is 25. If the median class interval is 20 –30 and the number of observations less than 20 is 45, then the frequency of median class is ______.
