Advertisements
Advertisements
प्रश्न
The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60°,
find the other angles.
उत्तर
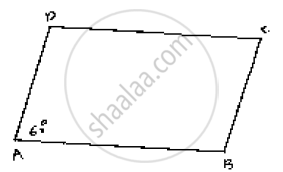
Given AB || CD
AD || BC
Since AB || CD and AD is the transversal
`∠`A + `∠`D = 180° [Co-interior angles are supplementary]
60° + `∠`D = 180°
`∠`D = 180° - 60°
`∠`D = 120°
Now, AD || BC and AB is the transversal
`∠`A + `∠`B = 180° [Co-interior angles are supplementary]
60° + `∠`B = 180°
`∠`B = 180° - 60° = 120°
Hence `∠`A = `∠`C = 60°
ÐB = ÐD =120°
APPEARS IN
संबंधित प्रश्न
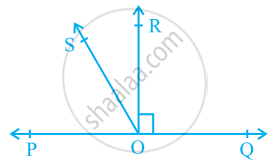
In the given figure, POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ∠ROS = `1/2` (∠QOS − ∠POS).
Write the complement of the following angles.
90°
Write the supplement of the following angles.
132°
An angle is equal to 8 times its complement. Determine its measure.
In the given below Fig, find x. Further find ∠BOC, ∠COD and ∠AOD .
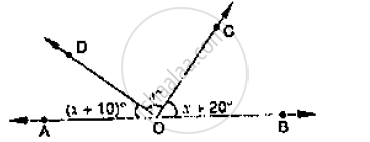
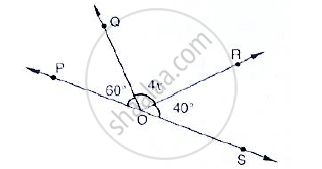
In the below fig, POS is a line, find x.
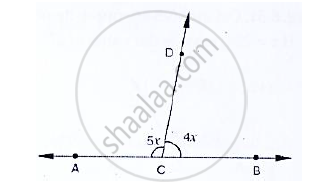
In the below fig, ACB is a line such that ∠DCA = 5x and ∠DCB = 4x. Find the value of x.
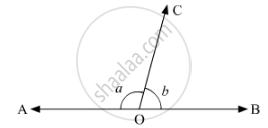
In Fig. 8.42, a is greater than b by one third of a right-angle. Find the values of a and b.
If below fig, if AB || CD and CD || EF, find ∠ACE.

In the below fig, `∠`1 = 60° and `∠`2 = `(2/3)^(rd)`of a right angle. Prove that l || m.