Advertisements
Advertisements
प्रश्न
Prove that the straight lines perpendicular to the same straight line are parallel to one
another.
उत्तर
Let AB and CD perpendicular to the Line MN
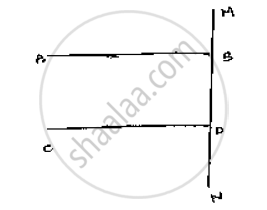
`∠`ABD = 90° [∵ AB ⊥ MN ] ......(i )
`∠`CON = 90° [ ∵ CD ⊥ MN] ......(ii)
Now,
`∠`ABD = `∠`CDN = 90° [From (i) and (ii)]
∴AB || CD, Since corresponding angles are equal.
APPEARS IN
संबंधित प्रश्न
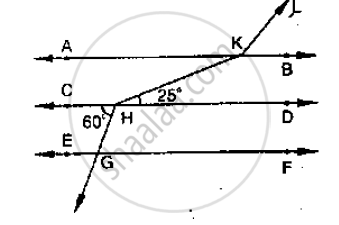
In the below fig, AB || CD || EF and GH || KL. Find `∠`HKL
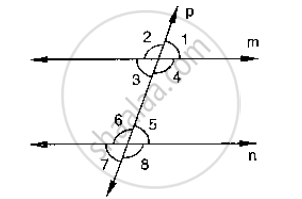
In the below fig, p is a transversal to lines m and n,`∠`2 = 120° and `a∠`5 = 60°. Prove that m || n.
In the below fig, arms BA and BC of `∠`ABC are respectively parallel to arms ED and EF of
`∠`DEF. Prove that `∠`ABC = ∠DEF.
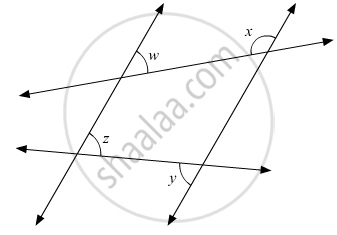
In the given figure, if l1 || l2, what is x + y in terms of w and z?
In the given figure, if l1 || l2, what is the value of y?

In the given figure, if AB || CD, then x =

In the given figure, if l || m, then x =

In the given figure, if lines l and m are parallel, then the value of x is

Two lines l and m are perpendicular to the same line n. Are l and m perpendicular to each other? Give reason for your answer.
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.