Advertisements
Advertisements
प्रश्न
In the given figure, if lines l and m are parallel, then the value of x is

विकल्प
35°
55°
65°
75°
उत्तर
The given figure is as follows with : l || m
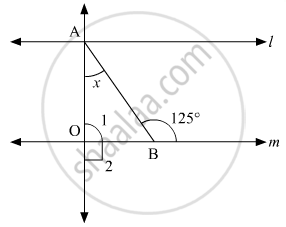
Also, ∠1 and ∠2 form a linear pair. Thus,
∠1 +∠2 = 180°
It is given that ∠2 = 90°, substituting this value , we get :
∠1 + 90° = 180°
∠1 = 180° - 190°
∠1 = 90° (i)
In a triangle, we know that, the exterior angle is equal to the sum of the interior opposite angle.
In ΔAOB:
x + ∠1 = 125°
x = 125° - ∠1
From equation (i):
x = 125° - 90°
x = 35°
APPEARS IN
संबंधित प्रश्न
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
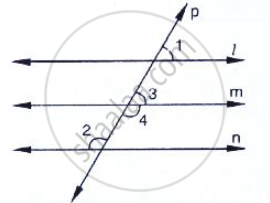
In the below fig, if l || m || n and `∠`1 = 60°, find `∠`2.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then the interior angles on the same side of the transversal are equal.
Fill in the blank in the following to make the statement true:
If two parallel lines are intersected by a transversal, then interior angles on the same
side of the transversal are _______
Fill in the blank :
If a transversal intersects a pair of lines in such a way that the sum of interior angles
on the same side of transversal is 180°, then the lines are _______.
If two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 2:3, then the measure of the larger angle is
AB and CD are two parallel lines. PQ cuts AB and CD at E and F respectively. EL is the bisector of ∠FEB. If ∠LEB = 35°, then ∠CFQ will be
In the following figure, if OP || RS, ∠OPQ = 110° and ∠QRS = 130°, then ∠PQR is equal to ______.

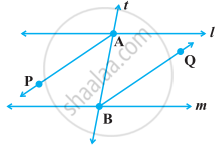
AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (Figure). Show that AP || BQ.
In the following figure, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°