Advertisements
Advertisements
प्रश्न
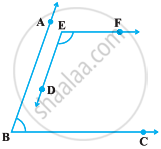
In the following figure, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°
उत्तर
It is given to us:
BA || ED
BC || EF
To show that: ∠ABC + ∠DEF = 180°
Let us extend DE to intersect BC at G and EF to intersect BA at H.
Then, the figure becomes
Since, BA || DE
⇒ BA || GE
We have two parallel lines BA and GE and BG is a transversal intersecting BA and GE at points B and G respectively.
⇒ ∠ABC = ∠EGC ...(i)
Also, BC || EF and GE is a transversal intersecting BC and EF at points G and E respectively.
⇒ ∠EGC = ∠HEG ...(ii)
Since GE is a ray standing on the line HF.
By linear pair axiom,
∠HEG + ∠GEF = 180°
⇒ ∠EGC + ∠GEF = 180° ...[From equation (ii)]
⇒ ∠ABC + ∠GEF = 180°
⇒ ∠ABC + ∠DEF = 180°
Hence, proved.
APPEARS IN
संबंधित प्रश्न
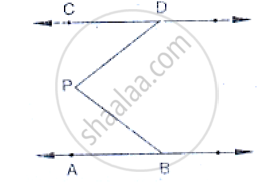
In the below fig, lines AB and CD are parallel and P is any point as shown in the figure.
Show that` ∠`ABP +` ∠`CDP = ∠DPB.
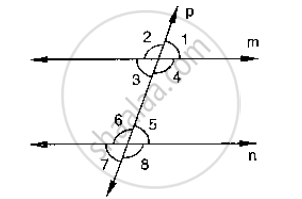
In the below fig, p is a transversal to lines m and n,`∠`2 = 120° and `a∠`5 = 60°. Prove that m || n.
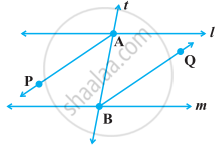
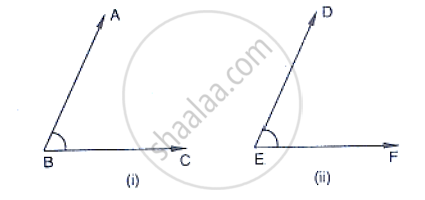
In the below fig, arms BA and BC of `∠`ABC are respectively parallel to arms ED and EF of
`∠`DEF. Prove that `∠`ABC = ∠DEF.
Fill in the blank in the following to make the statement true:
If two parallel lines are intersected by a transversal, then interior angles on the same
side of the transversal are _______
In the given figure, if AB || CD, then x =

In the given figure, if lines l and m are parallel lines, then x =

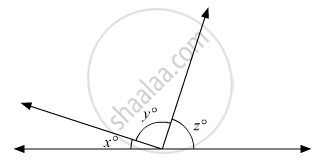
In the given figure, if \[\frac{y}{x} = 5\] and \[\frac{z}{x} = 4\] then the value of x isc
In the following figure, find the value of x for which the lines l and m are parallel.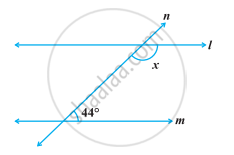
Two lines l and m are perpendicular to the same line n. Are l and m perpendicular to each other? Give reason for your answer.
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.