Advertisements
Advertisements
प्रश्न
In the following figure, DE || QR and AP and BP are bisectors of ∠EAB and ∠RBA, respectively. Find ∠APB.

उत्तर
Given, DE || QR and AP and PB are the bisectors of ∠EAB and ∠RBA, respectively.
We know that, the interior angles on the same side of transversal are supplementary.
∴ ∠EAB + ∠RBA = 180°
⇒ `1/2 ∠ EAB + 1/2 ∠RBA = (180^circ)/2` ...[Dividing both sides by 2]
⇒ `1/2 ∠EAB + 1/2 ∠RBA = 90^circ` ...(i)
Since, AP and BP are the bisectors of ∠EAB and ∠RBA, respectively.
∴ `∠BAP = 1/2 ∠EAB` ...(ii)
And `∠ABP = 1/2 ∠RBA` ...(iii)
On adding equations (ii) and (iii), we get
`∠BAP + ∠ABP = 1/2 ∠EAB + 1/2 ∠RBA`
From equation (i),
⇒ ∠BAP + ∠ABP = 90°
In ΔAPB, ∠BAP + ∠ABP + ∠APB = 180° ...[Sum of all angles of a triangle is 180°]
⇒ 90° + ∠APB = 180° ...[From equation (iv)]
⇒ ∠APB = 180° – 90° = 90°
APPEARS IN
संबंधित प्रश्न
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

Find the value of the unknown x in the following diagram:
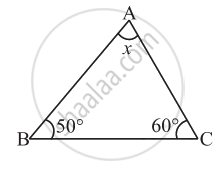
Find the value of the unknown x in the following diagram:

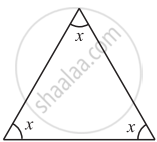
Find the value of the unknown x in the following diagram:
In the following triangle, find the value of x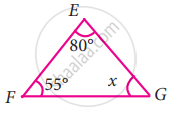
In a right angled triangle MNO, ∠N = 90°, MO is extended to P. If ∠NOP = 128°, find the other two angles of ∆MNO
If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
If one angle of a triangle is equal to the sum of other two, then the measure of that angle is ______.
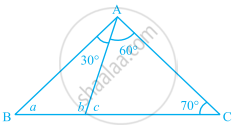
In the given figure, find the values of a, b and c.
In the given figure, if ST = SU, then find the values of x and y.

