Advertisements
Advertisements
प्रश्न
In the given figure, if l1 || l2, what is the value of y?

विकल्प
100°
120°
135°
150°
उत्तर
Given figure is as follows:
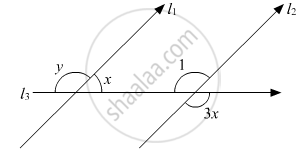
It is given that l1 || l2.
∠1 and 3x are vertically opposite angles, which must be equal, that is,
∠1 = 3x (i)
Also, ∠1and x are consecutive interior angles.
Theorem states: If a transversal intersects two parallel lines, then each pair of consecutive interior angles are supplementary.
Thus,
∠1 + x = 180°
From equation (i), we get:
`3x + x = 180°`
`4x = 180°`
` x = 180°/4`
`x = 45°`
x and y form a linear pair. Therefore, their sum must be supplementary.
Thus,
y + x =180°
Substituting, x = 45° in equation above, we get:
y + 45° = 180°
y = 180° - 45°
y = 135°
APPEARS IN
संबंधित प्रश्न
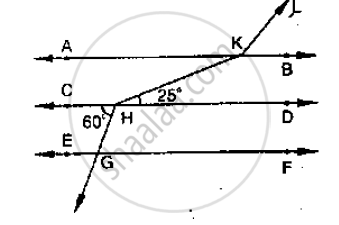
In the below fig, AB || CD || EF and GH || KL. Find `∠`HKL
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
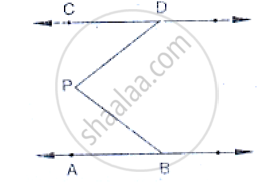
In the below fig, lines AB and CD are parallel and P is any point as shown in the figure.
Show that` ∠`ABP +` ∠`CDP = ∠DPB.
Which of the following statement are true and false ? Give reason.
If two parallel lines are intersected by a transversal, then alternate interior angles are equal.
In the given figure, PQ || RS, ∠AEF = 95°, ∠BHS = 110° and ∠ABC = x°. Then the value of x is

In the given figure, if lines l and m are parallel, then x =
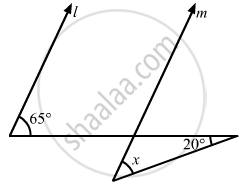
In the given figure, if AB || CD, then x =

In the given figure, if lines l and m are parallel lines, then x =

In the following figure, if OP || RS, ∠OPQ = 110° and ∠QRS = 130°, then ∠PQR is equal to ______.

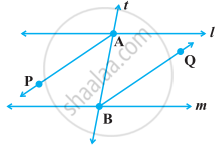
AP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (Figure). Show that AP || BQ.