Advertisements
Advertisements
प्रश्न
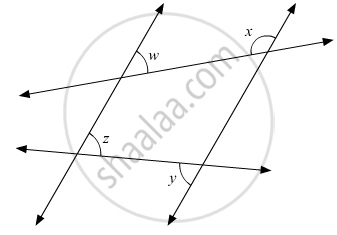
In the given figure, if l1 || l2, what is x + y in terms of w and z?
विकल्प
180° − w + z
180° + w − z
180° - w − z
180° + w + z
उत्तर
The figure is given below:

Since, y and z are alternate interior opposite angles. Therefore, these must be equal.
y = z (i)
Also x and w are consecutive interior angles.
Theorem states: If a transversal intersects two parallel lines, then each pair of consecutive interior angles are supplementary.
Therefore,
x +w = 180° (ii)
On adding equation (i) and (iii) , we get :
x + y + w = 180°
x + y = 180° + z - w
APPEARS IN
संबंधित प्रश्न
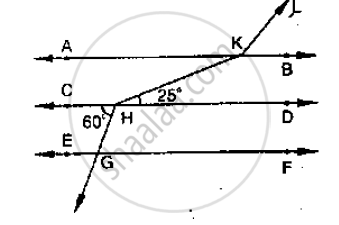
In the below fig, AB || CD || EF and GH || KL. Find `∠`HKL
If two straight lines are perpendicular to the same line, prove that they are parallel to each
other.
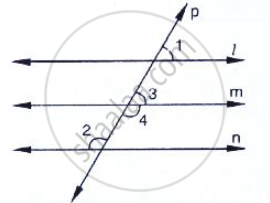
In the below fig, if l || m || n and `∠`1 = 60°, find `∠`2.
Fill in the blank :
If a transversal intersects a pair of lines in such a way that the sum of interior angles
on the same side of transversal is 180°, then the lines are _______.
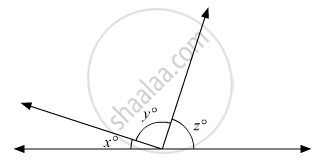
In the given figure, if l || m, then x =

In the given figure, if \[\frac{y}{x} = 5\] and \[\frac{z}{x} = 4\] then the value of x isc
In the following figure, bisectors AP and BQ of the alternate interior angles are parallel, then show that l ||m.
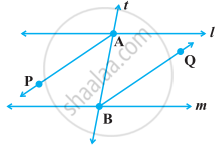
In the following figure, BA || ED and BC || EF. Show that ∠ABC = ∠DEF
[Hint: Produce DE to intersect BC at P (say)].
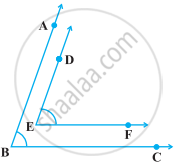
In the following figure, BA || ED and BC || EF. Show that ∠ABC + ∠DEF = 180°
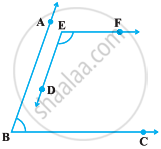
A transversal intersects two parallel lines. Prove that the bisectors of any pair of corresponding angles so formed are parallel.
