Advertisements
Advertisements
प्रश्न
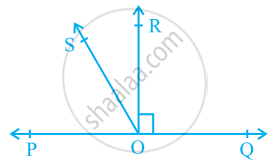
In the given figure, POQ is a line. Ray OR is perpendicular to line PQ. OS is another ray lying between rays OP and OR. Prove that ∠ROS = `1/2` (∠QOS − ∠POS).
उत्तर
It is given that OR ⊥ PQ
∴ ∠POR = 90°
⇒ ∠POS + ∠SOR = 90°
∠ROS = 90° − ∠POS …(1)
∠QOR = 90° ...(As OR ⊥ PQ)
∠QOS − ∠ROS = 90°
∠ROS = ∠QOS − 90° …(2)
On adding equations (1) and (2), we obtain
2 ∠ROS = ∠QOS − ∠POS
∴ ∠ROS = `1/2` (∠QOS − ∠POS)
APPEARS IN
संबंधित प्रश्न
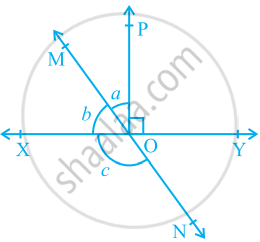
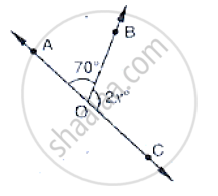
In the given figure, lines XY and MN intersect at O. If ∠POY = 90° and a : b = 2 : 3, find c.
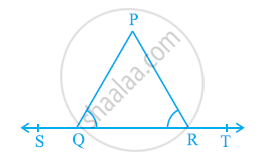
In the given figure, ∠PQR = ∠PRQ, then prove that ∠PQS = ∠PRT.
Write the supplement of the following angles .
138°
If the angles (2x −10)° and (x − 5)° are complementary angles, find x.
An angle is 14° more than its complementary angle. What is its measure?
How many pairs of adjacent angles, in all, can you name in below fig.?
In the below fig, AOC is a line, find x.
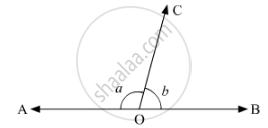
In Fig. 8.42, a is greater than b by one third of a right-angle. Find the values of a and b.
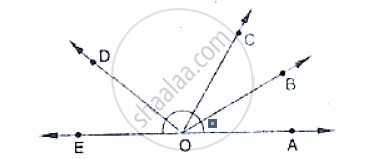
AB, CD and EF are three concurrent lines passing through the point O such that OF bisects
∠BOD. If ∠BOF = 35°, find ∠BOC and ∠AOD.
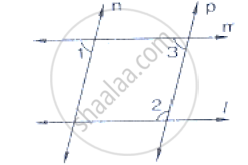
In the below fig, if l || m, n || p and ∠1 = 85°, find `∠`2.