Advertisements
Advertisements
प्रश्न
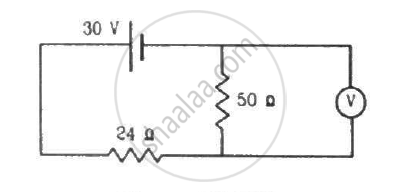
The voltmeter shown in the figure reads 18 V across the 50 Ω resistor. Find the resistance of the voltmeter.
उत्तर
Let R be the resistance of the voltmeter. For the given circuit, 50 Ω and R are in parallel. The equivalent resistance of these two resistors,
\[R_{eqv} = \frac{50R}{50 + R}\]
This equivalent resistance is now in series with the 24 Ω resistor. The effective resistance of the circuit,
\[R_{eff} = \left( \frac{50R}{50 + R} + 24 \right) \Omega\]
Current through the circuit,
\[i = \frac{30}{R_{eff}}\]
Voltage across the 24 Ω resistor, V1 = \[i \times 24 = \frac{30}{R_{eff}} \times 24 V\]
Voltage across the 50 Ω resistor, V2 = \[30 - V_1 = 30 - \frac{30}{R_{eff}} \times 24 V ............\left(\because V_1 + V_2 = 30\right)\]
It is given that V2 = 18 V
\[\Rightarrow 18 = 30 - \frac{30}{R_{eff}} \times 24\]
\[ \Rightarrow 18 = 30 - \frac{30}{\left( \frac{50R}{50 + R} + 24 \right)} \times 24\]
\[ \Rightarrow \frac{30}{\left( \frac{74R + 1200}{50 + R} \right)} \times 24 = 30 - 18\]
\[ \Rightarrow \frac{30}{\left( \frac{74R + 1200}{50 + R} \right)} = \frac{12}{24} = \frac{1}{2}\]
\[ \Rightarrow 60\left( 50 + R \right) = \left( 74R + 1200 \right)\]
\[ \Rightarrow R \approx 130 \Omega\]
APPEARS IN
संबंधित प्रश्न
Two resistances R and 2R are connected in parallel in an electric circuit. The thermal energy developed in R and 2R are in the ratio _______________ .
A uniform wire of resistance 50 Ω is cut into 5 equal parts. These parts are now connected in parallel. The equivalent resistance of the combination is ______________ .
A bulb is made using two filaments. A switch selects whether the filaments are used individually or in parallel. When used with a 15 V battery, the bulb can be operated at 5 W, 10 W or 15 W. What should be the resistances of the filaments?
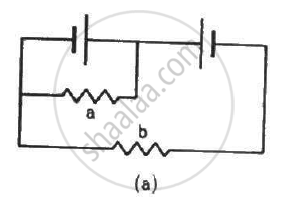
Each of the resistors shown in the figure has a resistance of 10 Ω and each of the batteries has an emf of 10 V. Find the currents flowing through the resistors a and bin the two circuits.

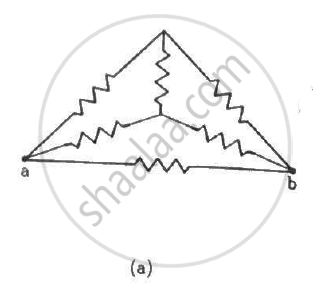
Find the equivalent resistance of the circuits shown in the figure between the points a and b. Each resistor has resistance r.

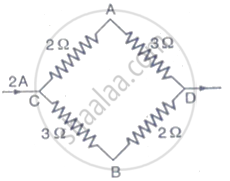
Find the current measured by the ammeter in the circuit shown in the figure.

The emf ε and the internal resistance r of the battery, shown in the figure, are 4.3 V and 1.0 Ω respectively. The external resistance R is 50 Ω. The resistances of the ammeter and voltmeter are 2.0 Ω and 200 Ω respectively. (a) Find the readings of the two meters. (b) The switch is thrown to the other side. What will be the readings of the two meters now?

A current of 2 A flows in conductors as shown. The potential difference VA- VB will be ________.
In parallel combination of n cells, we obtain ______.
Three resistors having values R battery. Suppose R1 carries a current of 2.0 A, R ohms, and R3 dissipates 6.0 watts of power. Then the voltage across R is ______.
Assertion (A): To increase the range of an ammeter, we must connect a suitable high resistance in series to it.
Reason (R): The ammeter with increased range should have high resistance.
Select the most appropriate answer from the options given below:
How many times will the resistance of an identical conductor be increase, if the parallel resistance be change to series one?
The equivalent resistance of resistors connected in series is always ______
The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section and the same material is 0.25 Ω. What will be the effective resistance if they are connected in series?
The effective resistance of a parallel connection that consists of four wires of equal length, equal area of cross-section and same material is 0.25 Ω. What will be the effective resistance if they are connected in series?
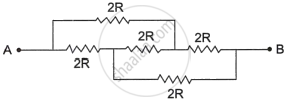
- Assertion (A): The equivalent resistance between points A and B in the given networks is 2R.
- Reason (R): All the resistors are connected in parallel.
Three bulbs B1 (230V, 40W), B2 (230V, 60W) and B3 (230V, 100W) are connected in series to a 230V supply. Which bulb glows the brightest?
