Question

Three equal masses m are placed at the three corners of an equilateral triangle of side a. Find the force exerted by this system on another particle of mass m placed at (a) the mid-point of a side, (b) at the centre of the triangle.

Answer 1

(a) Consider that mass 'm' is placed at the midpoint O of side AB of equilateral triangle ABC.

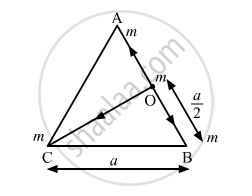
AO = BO = \[\frac{a}{2}\]
Then \[\overrightarrow{F}_{OA} = \frac{4G m^2}{a^2}\] along OA

Also, \[\overrightarrow{F}_{OB} = \frac{4 G m^2}{a^2}\]along OB

OC = \[\frac{\sqrt{3}a}{2}\]

\[\overrightarrow{F}_{OC} = \frac{4 G m^2}{\left\{ \left( 3 \right) a^2 \right\}} = \frac{4G m^2}{3 a^2}\] along OC

The net force on the particle at O is \[\overrightarrow{F} = \overrightarrow{F}_{OA} + \overrightarrow{F}_{OB} + \overrightarrow{F}_{OC}\]

Since equal and opposite forces cancel each other, we have :

\[\overrightarrow{F} = \overrightarrow{F}_{OC} = \frac{4 G m^2}{\left\{ \left( 3 \right) a^2 \right\}} = \frac{4G m^2}{3 a^2}\] along OC.

(b) If the particle placed at O (centroid)

All the forces are equal in magnitude but their directions are different as shown in the figure.
Equal and opposite forces along OM and ON cancel each other.
i.e., \[F\cos30^\circ= F\cos30^\circ\]

∴ Resultant force \[= F - 2F\sin30 = 0\]