Advertisements
Advertisements
प्रश्न
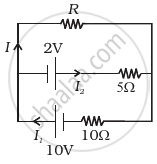
Two cells of voltage 10V and 2V and internal resistances 10Ω and 5Ω respectively, are connected in parallel with the positive end of 10V battery connected to negative pole of 2V battery (Figure). Find the effective voltage and effective resistance of the combination.
Advertisements
उत्तर
In this problem first, we are applying Kirchhoff's junction rule at c, I1 = I + I2
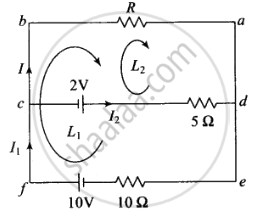
Applying Kirchhoff's voltage law in loop (e – f – b – a – e) loop L1 outer loop, then we get
10 – IR – 10l1 = 0
10 = IR + 10l1 ......(i)
Applying Kirchhoff's voltage law in loop (c – b – a – d – c) loop L2, we get
– 2 – IR + 5I2 = 0
2 = 5I2 – RI
As we know, I1 = I + I2 then
I2 = I1 – I
So the above equation can be written as
2 = 5(I1 – I) – RI
or 4 = 10I1 – 10I – 2RI ......(ii)
Subtracting equations (ii) from (i), we get
⇒ 6 = 3RI + 10I
2 = `I(R + 10/3)`
Also, the external resistance is R. The Ohm's law states that V = I(R + Reff)
On comparing, we have V = 2V and effective internal resistance `(R_("eff")) = (10/3)Ω`
Since, the equivalent internal resistance (Reff) of two cells is `(10/3)Ω`, being the parallel combination of 5Ω and 10Ω. The equivalent circuit is given below:
APPEARS IN
संबंधित प्रश्न
Kirchhoff's voltage law and current law are respectively in accordance with the conservation of .................................. .
- charge and momentum
- charge and energy
- energy and charge
- energy and momentum
Given the resistances of 1 Ω, 2 Ω, 3 Ω, how will be combine them to get an equivalent resistance of 6 Ω?
Given the resistances of 1 Ω, 2 Ω, 3 Ω, how will be combine them to get an equivalent resistance of (6/11) Ω?
Determine the equivalent resistance of networks shown in Fig.
Calculate the value of the resistance R in the circuit shown in the figure so that the current in the circuit is 0.2 A. What would b the potential difference between points A and B?
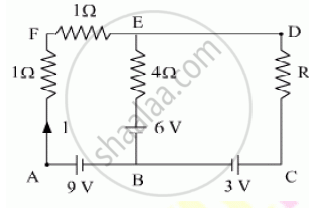
In the given circuit, assuming point A to be at zero potential, use Kirchhoff’s rules to determine the potential at point B.
Find the circuit in the three resistors shown in the figure.
Consider the circuit shown in the figure. Find (a) the current in the circuit (b) the potential drop across the 5 Ω resistor (c) the potential drop across the 10 Ω resistor (d) Answer the parts (a), (b) and (c) with reference to the figure.
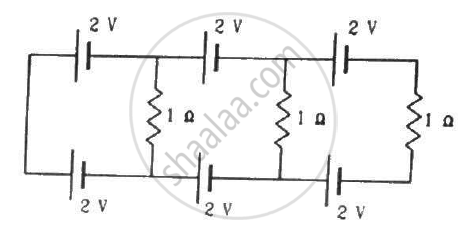
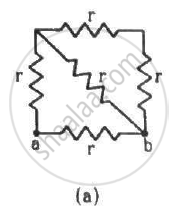
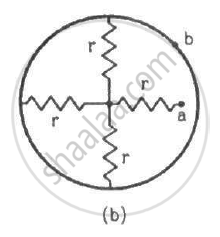
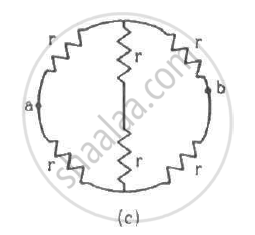
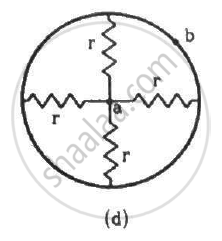
Twelve wires, each of equal resistance r, are joined to form a cube, as shown in the figure. Find the equivalent resistance between the diagonally-opposite points a and f.
Two unequal resistances, R1 and R2, are connected across two identical batteries of emf ε and internal resistance r (see the figure). Can the thermal energies developed in R1 and R2 be equal in a given time? If yes, what will be the condition?
On which conservation principle is Kirchoff's Second Law of electrical networks based?
State the principle of potentiometer.
State and explain Kirchhoff’s rules.
Obtain the condition for bridge balance in Wheatstone’s bridge.
How the emf of two cells are compared using potentiometer?
In a potentiometer arrangement, a cell of emf 1.25 V gives a balance point at 35 cm length of the wire. If the cell is replaced by another cell and the balance point shifts to 63 cm, what is the emf of the second cell?
The instrument for the accurate measurement of the e.m.f of a cell is ______.
While measuring the length of the rod by vernier callipers, the reading on the main scale is 6.4 cm and the eight divisions on vernier is in line with marking on the main scale division. If the least count of callipers is 0.01 and zero error - 0.04 cm, the length of the rod is ______.
Kirchhoff’s junction rule is a reflection of ______.
- conservation of current density vector.
- conservation of charge.
- the fact that the momentum with which a charged particle approaches a junction is unchanged (as a vector) as the charged particle leaves the junction.
- the fact that there is no accumulation of charges at a junction.
