Advertisements
Advertisements
प्रश्न
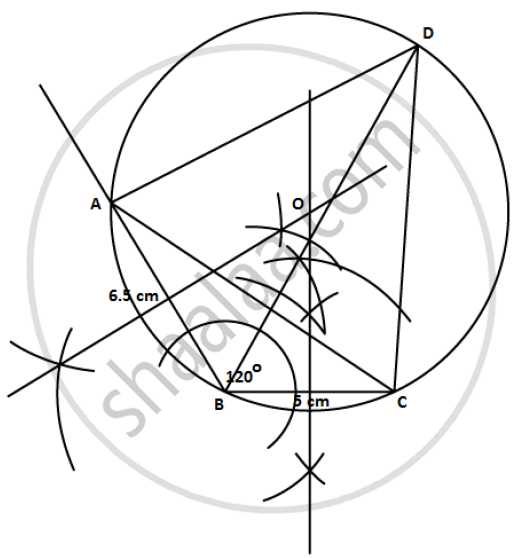
Using ruler and compass only, construct a ΔABC such that BC = 5 cm and AB = 6.5
cm and ∠ABC = 120°
1) Construct a circum-circle of ΔABC
2) Construct a cyclic quadrilateral ABCD, such that D is equidistant from AB and BC.
उत्तर
Steps of construction:
1) Draw a line segment BC of length 5 cm.
2) At B, draw a ray BX making an angle of 120° with BC.
3) With B as centre and radius 6.5 cm, draw an arc to cut the ray BX at A. Join AC.
ΔABC will be obtained
4) Draw the perpendicular bisectors of AB and BC to meet at point O.
5) With O as centre and radius OA, draw a circle. The circle will circumscribe ΔABC
6) Draw the angle bisector of ∠ABC
7) The angle bisector of ABC and let it meet circle at point D.
8) Join AD and DC to obtain the required cyclic quadrilateral ABCD such that point D is equidistant from AB and BC.
APPEARS IN
संबंधित प्रश्न
Construct a regular hexagon of side 5 cm. Hence construct all its lines of symmetry and name them.
Use ruler and compasses only for the following questions. All constructions lines and arcs must be clearly shown
Construct a ABC in which BC = 6.5 cm, ABC = 60°, AB = 5 cm.
Constuct a triangle ABC with AB = 5.5 cm, AC = 6 cm and ∠BAC = 105°. Hence:
- Construct the locus of point equdistant from BA and BC.
- Construct the locus of points equidistant from B and C.
- Mark the point which satisfies the above two loci as P. Measure and write the length of PC.
Using ruler and compasses only, construct Δ ABC in which BC=7.5 cm, ∠ ABC = 60° and AC - AB= 1.5 cm. Inscribe a circle in the Δ ABC and measure its radius.
Draw a circle with radius 3 cm and inscribe an equilateral triangle in it.
Perpendicular bisectors of the sides AB and AC of a triangle ABC meet at O.
Does the perpendicular bisector of BC pass through O?
Construct a triangle whose sides are 4.4 cm, 5.2 cm, and 7.1 cm. Construct its circumcircle. Write also the steps of construction.
Use ruler and compasses only for this question:
(i) Construct A ABC, where AB = 3.5 cm, BC = 6 cm and ∠ ABC = 60°.
(ii) Construct the locus of points inside the triangle which are equidistant from BA and BC.
(iii) Construct the locus of points inside the triangle which are equidistant from B and C.
(iv) Mark the point P which is equidistant from AB, BC, and also equidistant from B and C. Measure and record the length of PB.
(i) Construct a triangle ABC, in which AB = 5.0 cm, BC = 3.5 cm and ∠ ABC = `67 1/2°`
( Use a pair of compasses and ruler only.)
(ii) Construct a circle to touch AB at B and it pass though C.
Ruler and compasses only may be used in this question. All constructions lines and arcs must be clearly shown, and the be sufficient length and clarity to permit assessment:
(i) Construct a triangle ABC, in which AB = 9 cm, BC = 10 cm and angle ABC = 45°.
(ii) Draw a circle, with center A and radius 2.5 cm. Let it meet AB at D.
(iii) Construct a circle to touch the circle with center A externally at D and also to touch the line BC.
