Advertisements
Advertisements
प्रश्न
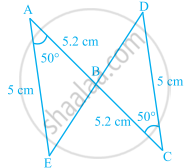
Using the given figure, prove that the triangles are congruent. Can you conclude that AC is parallel to DE
उत्तर
In ∆ABC and ∆EBD,
AB = EB
BC = BD
∠ABC = ∠EBD ...[∵ Vertically opposite angles]
By SAS congruency criteria ∆ABC ≅ ∆EBD.
We know that corresponding parts of congruent triangles are congruent.
∴ ∠BCA ≅ ∠BDE and ∠BAC ≅ ∠BED
∠BCA ≅ ∠BDE means that alternate interior angles are equal if CD is the transversal to lines AC and DE.
Similarly, if AE is the transversal to AC and DE,
we have ∠BAC ≅ ∠BED
Again interior opposite angles are equal.
We can conclude that AC is parallel to DE.
APPEARS IN
संबंधित प्रश्न
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, by using the SAS congruence rule. If the triangles are congruent, write them in symbolic form.
∆ABC, AB = 7 cm, BC = 5 cm, ∠B = 50°.
∆DEF, DE = 5 cm, EF = 7 cm, ∠E = 50°.
In the given figure, ΔBCD is isosceles with base BD and ∠BAE ≡∠DEA. Prove that AB ≡ ED
In the figure, given that ∠1 = ∠2 and ∠3 ≡ ∠4. Prove that ∆MUG ≡ ∆TUB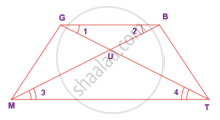
Construct a triangle ABC with given conditions.
AB = 7 cm, AC = 6.5 cm and ∠A = 120°
Construct a triangle PQR with given conditions.
∠P = 60°, ∠R = 35° and PR = 7.8 cm
In the given figure, ΔPQR ≅ Δ ______.

In the given figures, two triangles are congruent by RHS.
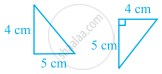
In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆PQR: PQ = 3.5 cm, QR = 4.0 cm, ∠Q = 60°
∆STU: ST = 3.5 cm, TU = 4 cm, ∠T = 60°
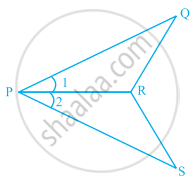
In the given figure, PO = PS and ∠1 = ∠2.
- Is ∆PQR ≅ ∆PSR? Give reasons.
- Is QR = SR? Give reasons.