Question

While driving along an unbanked circular road, a two-wheeler rider has to lean with the vertical. Why is it so? With what angle the rider has to lean? Derive the relevant expression. Why such a leaning is not necessary for a four wheeler?

Answer 1

(i) When a bicyclist takes a turn along an unbanked road, the force of friction `vec"f"_"s"` provides the centripetal force; the normal reaction of the road `vec"N"` is vertically up. If the bicyclist does not lean inward, there will be an unbalanced outward torque about the centre of gravity, f_s·h, due to the friction force that will topple the bicyclist outward. The bicyclist must lean inward to counteract this torque (and not to generate a centripetal force) such that the opposite inward torque of the couple formed by `vec"N"` and the weight `vec"g"`, mg.a = f_s.h₁ 

(a)	(b)

A bicyclist taking a turn to his left on a level road

(ii) Since the force of friction provides the centripetal force,

f_s = `"mv"^2/"r"`

If the cyclist leans from the vertical by an angle θ, the angle between `vec"N"` and `vec"F"` 

tan θ = `"f"_"s"/"N" = ("mv"^2//"r")/"mg" = "v"^2/"gr"`

Hence, the cyclist must lean by an angle

θ = tan^-1 `("v"^2/"gr")`

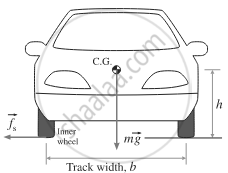
(iii) When a car takes a turn along a level road, apart from the risk of skidding off outward, it also has a tendency to roll outward due to an outward torque about the Centre of gravity due to the friction force. But a car is an extended object with four wheels. So, when the inner wheels just get lifted above the ground, it can be counterbalanced by a restoring torque of the couple formed by the normal reaction (on the outer wheels) and the weight. Consider a car of mass m taking a turn of radius r along a level road. As seen from an inertial frame of reference, the forces acting on the car are:

(1) the lateral limiting force of static friction `vec"f"_"s"` on the wheels – acting along the axis of the wheels and towards the center of the circular path which provides the necessary centripetal force.

(2) the weight `vec"m""g"` acting vertically downwards at the centre of gravity (C.G.)

(3) the normal reaction `vec"N"` of the road on the wheels, upwards effectively at the C.G. Since maximum centripetal force = limiting force of static friction,

`"ma"_"r" = "mv"^2/"r" = "f"_"s"`    ...(1)

In a simplified rigid-body vehicle model, we consider only two parameters – the height h of the C.G. above the ground and the average distance b between the left and right wheels called the track width.

(a)	(b)	(c)

Rolling tendency of a vehicle negotiating a bend on a level road

The friction force `vec"f"_"s"` on the wheels produces a torque `tau_"t"` that tends to overturn/rollover the car about the outer wheel in the above figure (b). Rotation about the front-to-back axis is called roll.

`tau_"t" = "f"_"s"."h" = ("mv"^2/"r")"h"`    ...(2)

When the inner wheel just gets lifted above the ground, the normal reaction `vec"N"` of the road acts on the outer wheels but the weight continues to act at the C.G. Then, the couple formed by the normal reaction and the weight produces a opposite torque `tau_"r"` which tends to restore the car back on all four wheels in the above figure (b).

`tau_"r" = "mg"."b"/2`     ....(3)

The car does not topple as long as the restoring torque `tau_"r"` counterbalances the toppling torque `tau_"r"`.
Thus, to avoid the risk of rollover, the maximum speed that the car can have is given by

`("mv"^2/"r")"h" = "mg"."b"/2`

`therefore "v"_"max" = sqrt("rbg"/"2h")`  ...(4)

Thus, vehicle tends to roll when the radial acceleration reaches a point where inner wheels of the four-wheeler are lifted off of the ground and the vehicle is rotated outward. A rollover occurs when the gravitational force `vec"mg"` passes through the pivot point of the outer wheels, i.e., the C.G. is above the line of contact of the outer wheels. Equation (3) shows that this maximum speed is high for a car with larger track width and lower center of gravity.