Advertisements
Advertisements
प्रश्न
Write the coefficient of the polynomial p(z) = z5 − 2z2 + 4.
उत्तर
We have to find the co-efficient of the polynomial p(z) = z5 − 2z2 + 4.
Co-efficient of `z^5 = 1`
Co-efficient of `z ^4 = 0`
Co-efficient of `z^3 = 0 `
Co-efficient of ` z^2 = -2 `
Co-efficient of z = 0
Constant term = 4
Hence, the co-efficient of `z^5,z^4,z^3,z^2,z` and constant term is `1,0,0 -2,0,4`
APPEARS IN
संबंधित प्रश्न
Classify the following polynomials as linear, quadratic, cubic and biquadratic polynomials
`7t^4+4t^3+3t-2`
State division algorithm for polynomials.
If f(x) = x3 + x2 − ax + b is divisible by x2 − x write the value of a and b.
If a − b, a and b are zeros of the polynomial f(x) = 2x3 − 6x2 + 5x − 7, write the value of a.
If (x + a) is a factor of 2x2 + 2ax + 5x + 10, find a.
If α, β are the zeros of a polynomial such that α + β = −6 and αβ = −4, then write the polynomial.
Divide. Write the quotient and the remainder.
(6x5 − 4x4 + 8x3 + 2x2) ÷ 2x2
Let the polynomials be
(A) −13q5 + 4q2 + 12q
(B) (x2 + 4)(x2 + 9)
(C) 4q8 – q6 + q2
(D) `– 5/7 y^12 + y^3 + y^5`
Then ascending order of their degree is
Case Study -1

The figure given alongside shows the path of a diver, when she takes a jump from the diving board. Clearly it is a parabola.
Annie was standing on a diving board, 48 feet above the water level. She took a dive into the pool. Her height (in feet) above the water level at any time ‘t’ in seconds is given by the polynomial h(t) such that h(t) = -16t2 + 8t + k.
A polynomial q(t) with sum of zeroes as 1 and the product as -6 is modelling Anu’s height in feet above the water at any time t( in seconds). Then q(t) is given by ______.
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.

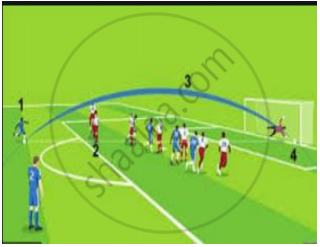
Observe the following graph and answer.
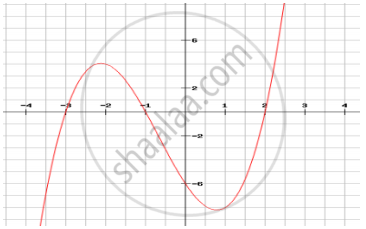
In the above graph, how many zeroes are there for the polynomial?
