Advertisements
Advertisements
प्रश्न
A 4 µF capacitor is charged by a 200 V supply. It is then disconnected from the supply and is connected to another uncharged 2 µF capacitors. How much electrostatic energy of the first capacitor is lost in the form of heat and electromagnetic radiation?
उत्तर
Capacitance of a charged capacitor, `"C"_1 = mu"F" = 4 xx 10^-6 "F"`
Supply voltage, V1 = 200 V
Electrostatic energy stored in C1 is given by,
`"E"_1 = 1/2"C"_1"V"_1^2`
= `1/2 xx 4 xx 10^-6 xx (200)^2`
= `8 xx 10^-2 "J"`
Capacitance of an uncharged capacitor, `"C"_2 = 2mu"F" = 2 xx 10^-6 "F"`
When C2 is connected to the circuit, the potential acquired by it is V2.
According to the conservation of charge, the initial charge on capacitor C1 is equal to the final charge on capacitors, C1 and C2.
∴ `"V"_2("C"_1 + "C"_2) = "C"_1"V"_1`
`"V"_2 xx (4 + 2) xx 10^-6 = 4 xx 10^-6 xx 200`
`"V"_2 = 400/3` V
Electrostatic energy for the combination of two capacitors is given by,
`"E"_2 = 1/2("C"_1 + "C"_2)"V"_2^2`
= `1/2(2 + 4)xx 10^-6 xx (400/3)^2`
= `5.33 xx 10^-2` J
Hence, amount of electrostatic energy lost by capacitor C1
= E1 − E2
= 0.08 − 0.0533
= 0.0267
= 2.67 × 10−2 J
APPEARS IN
संबंधित प्रश्न
A spherical conductor of radius 12 cm has a charge of 1.6 × 10−7 C distributed uniformly on its surface. What is the electric field
- inside the sphere
- just outside the sphere
- at a point 18 cm from the centre of the sphere?
A spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell?
(b) Is the electric field inside a cavity (with no charge) zero, even if the shell is not spherical, but has any irregular shape? Explain.
(a) Show that the normal component of electrostatic field has a discontinuity from one side of a charged surface to another given by
`(vec"E"_2 - vec"E"_1).hat"n" = sigma/in_0`
Where `hat"n"` is a unit vector normal to the surface at a point and σ is the surface charge density at that point. (The direction of `hat"n"` is from side 1 to side 2.) Hence show that just outside a conductor, the electric field is σ `hat"n"/in_0`
(b) Show that the tangential component of electrostatic field is continuous from one side of a charged surface to another.
[Hint: For (a), use Gauss’s law. For, (b) use the fact that work done by electrostatic field on a closed loop is zero.]
A 12 pF capacitor is connected to a 50 V battery. How much electrostatic energy is stored in the capacitor? If another capacitor of 6 pF is connected in series with it with the same battery connected across the combination, find the charge stored and potential difference across each capacitor.
Define electrostatic potential at a point. Write its S.I. unit. Three-point charges q1, q2 and q3 are kept respectively at points A, B, and C as shown in the figure, Derive the expression for the electrostatic potential energy of the system.

Electric-field magnitude 'E' at points inside and outside a positively charged spherical conductor having charge Q and a radius R are ______.
If R is the radius of a spherical conductor, Vm the dielectric strength, then the maximum electric-field magnitude to which it can be raised is ______.
The electrostatic potential on the surface of a charged conducting sphere is 100V. Two statements are made in this regard S1 at any point inside the sphere, electric intensity is zero. S2 at any point inside the sphere, the electrostatic potential is 100 V. Which of the following is a correct statement?
Which of the following statement is true?
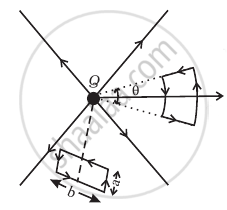
A test charge q is made to move in the electric field of a point charge Q along two different closed paths (Figure). First path has sections along and perpendicular to lines of electric field. Second path is a rectangular loop of the same area as the first loop. How does the work done compare in the two cases?
Consider a finite insulated, uncharged conductor placed near a finite positively charged conductor. The uncharged body must have a potential:
