Advertisements
Advertisements
प्रश्न
A candidate is required to answer 7 questions out of 12 questions, which are divided into two groups, each containing 6 questions. He is not permitted to attempt more than 5 questions from either group. Find the number of different ways of doing question
उत्तर
Total number of questions = 12
Number of questions in each group = 6
7 questions are to be attempted but not more than 5 questions from either group
∴ Total number of ways = 6C5 × 6C2 × 6C4 × 6C3 + 6C3 × 6C4 + 6C2 × 6C5
= 2[6C5 × 6C2 + 6C4 × 6C3]
= `2[6 xx (6.5)/(2.1) + (6*5*4*3)/(4*3*2*1) xx (6*5*4)/(3*2*1)]`
= 2[6 × 15 + 15 × 20]
= 2[90 + 300]
= 2 × 380
= 780
Hence, the total number of ways = 780
APPEARS IN
संबंधित प्रश्न
How many 3-digit even numbers can be formed from the digits 1, 2, 3, 4, 5, 6 if the digits can be repeated?
How many numbers between 100 and 1000 have 4 in the units place?
How many two letter words can be formed using letters from the word SPACE, when repetition of letters is allowed?
How many two letter words can be formed using letters from the word SPACE, when repetition of letters is not allowed?
How many three-digit numbers can be formed from the digits 0, 1, 3, 5, 6 if repetitions of digits are not allowed?
How many three-digit numbers can be formed using the digits 2, 3, 4, 5, 6 if digits can be repeated?
A letter lock contains 3 rings, each ring containing 5 different letters. Determine the maximum number of false trials that can be made before the lock is opened?
In a test, 5 questions are of the form 'state, true or false'. No student has got all answers correct. Also, the answer of every student is different. Find the number of students appeared for the test.
If numbers are formed using digits 2, 3, 4, 5, 6 without repetition, how many of them will exceed 400?
How many numbers formed with the digits 0, 1, 2, 5, 7, 8 will fall between 13 and 1000 if digits can be repeated?
Answer the following:
A hall has 12 lamps and every lamp can be switched on independently. Find the number of ways of illuminating the hall.
How many two-digit numbers can be formed using 1, 2, 3, 4, 5 without repetition of digits?
Four children are running a race:
In how many different ways could they finish the race?
How many three-digit numbers are there with 3 in the unit place?
with repetition
How many three-digit numbers are there with 3 in the unit place?
without repetition
How many numbers are there between 100 and 500 with the digits 0, 1, 2, 3, 4, 5? if the repetition of digits is not allowed
To travel from a place A to place B, there are two different bus routes B1, B2, two different train routes T1, T2 and one air route A1. From place B to place C there is one bus route say B1, two different train routes say T1, T2 and one air route A1. Find the number of routes of commuting from place A to place C via place B without using similar mode of transportation
How many numbers are there between 1 and 1000 (both inclusive) which are divisible neither by 2 nor by 5?
Find the value of 6!
Evaluate `("n"!)/("r"!("n" - "r")!)` when n = 6, r = 2
Evaluate `("n"!)/("r"!("n" - "r")!)` when for any n with r = 2
Find the value of n if (n + 1)! = 20(n − 1)!
In how many ways can this diagram be coloured subject to the following two conditions?
(i) Each of the smaller triangle is to be painted with one of three colours: red, blue or green.
(ii) No two adjacent regions have the same colour.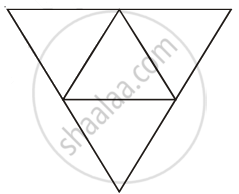
Eight chairs are numbered 1 to 8. Two women and 3 men wish to occupy one chair each. First the women choose the chairs from amongst the chairs 1 to 4 and then men select from the remaining chairs. Find the total number of possible arrangements.
If the letters of the word RACHIT are arranged in all possible ways as listed in dictionary. Then what is the rank of the word RACHIT?
Find the number of positive integers greater than 6000 and less than 7000 which are divisible by 5, provided that no digit is to be repeated.
The number of possible outcomes when a coin is tossed 6 times is ______.
Three letters can be posted in five letterboxes in 35 ways.
In a steamer there are stalls for 12 animals, and there are horses, cows and calves (not less than 12 each) ready to be shipped. They can be loaded in 312 ways.
There will be only 24 selections containing at least one red ball out of a bag containing 4 red and 5 black balls. It is being given that the balls of the same colour are identical.
