Advertisements
Advertisements
प्रश्न
A company manufactures two types of toys A and B. A toy of type A requires 5 minutes for cutting and 10 minutes for assembling. A toy of type B requires 8 minutes for cutting and 8 minutes for assembling. There are 3 hours available for cutting and 4 hours available for assembling the toys in a day. The profit is ₹ 50 each on a toy of type A and ₹ 60 each on a toy of type B. How many toys of each type should the company manufacture in a day to maximize the profit? Use linear programming to find the solution.
उत्तर
| Toy A | Toy B | Time in a day | |
| Cutting time | 5 min | 8 min | 180 min |
| Assembling time | 10 min | 8 min | 240 min |
| Profit | 50 | 60 | |
| Assumed quantity | x | y |
Profit function z = 50x + 60y
x ≥ 0, y ≥ 0
5x + 8y ≤ 180
10x + 8y ≤ 240 or 5x + 4y ≤ 120
5x + 8y = 180
| A | B | |
| x | 0 | 36 |
| y | 22.5 | 8 |
5x + 4y ≤ 120
| C | D | |
| x | 0 | 24 |
| y | 30 | 0 |
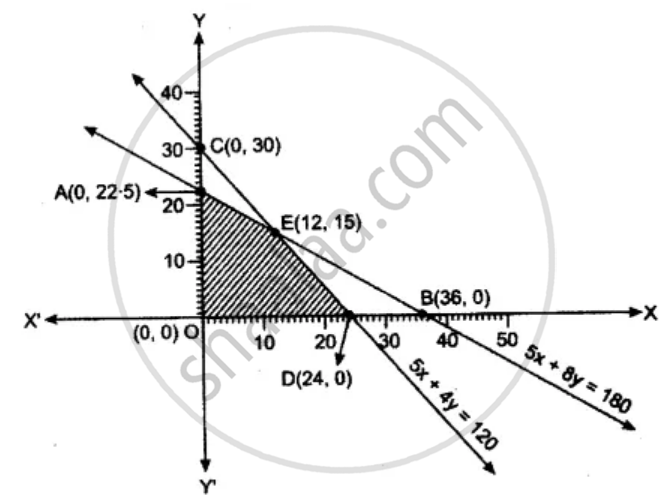
| Corner point | z = 50x + 60y |
| At O (0, 0) | 0 |
| At D (24, 0) | 1200 |
| At E (12, 15) | 1500 |
| At A (0, 22.5) | 1350 |
Hence, the maximum profit is Rs 1500 at E (12, 15).
APPEARS IN
संबंधित प्रश्न
A company is making two products A and B. The cost of producing one unit of products A and B are Rs 60 and Rs 80 respectively. As per the agreement, the company has to supply at least 200 units of product B to its regular customers. One unit of product A requires one machine hour whereas product B has machine hours available abundantly within the company. Total machine hours available for product A are 400 hours. One unit of each product A and B requires one labour hour each and total of 500 labour hours are available. The company wants to minimize the cost of production by satisfying the given requirements. Formulate the problem as a LPP.
Vitamins A and B are found in two different foods F1 and F2. One unit of food F1contains 2 units of vitamin A and 3 units of vitamin B. One unit of food F2 contains 4 units of vitamin A and 2 units of vitamin B. One unit of food F1 and F2 cost Rs 50 and 25 respectively. The minimum daily requirements for a person of vitamin A and B is 40 and 50 units respectively. Assuming that any thing in excess of daily minimum requirement of vitamin A and B is not harmful, find out the optimum mixture of food F1 and F2 at the minimum cost which meets the daily minimum requirement of vitamin A and B. Formulate this as a LPP.
A firm manufactures two products, each of which must be processed through two departments, 1 and 2. The hourly requirements per unit for each product in each department, the weekly capacities in each department, selling price per unit, labour cost per unit, and raw material cost per unit are summarized as follows:
| Product A | Product B | Weekly capacity | |
| Department 1 | 3 | 2 | 130 |
| Department 2 | 4 | 6 | 260 |
| Selling price per unit | Rs 25 | Rs 30 | |
| Labour cost per unit | Rs 16 | Rs 20 | |
| Raw material cost per unit | Rs 4 | Rs 4 |
The problem is to determine the number of units to produce each product so as to maximize total contribution to profit. Formulate this as a LPP.
An airline agrees to charter planes for a group. The group needs at least 160 first class seats and at least 300 tourist class seats. The airline must use at least two of its model 314 planes which have 20 first class and 30 tourist class seats. The airline will also use some of its model 535 planes which have 20 first class seats and 60 tourist class seats. Each flight of a model 314 plane costs the company Rs 100,000 and each flight of a model 535 plane costs Rs 150,000. How many of each type of plane should be used to minimize the flight cost? Formulate this as a LPP.
A firm has to transport at least 1200 packages daily using large vans which carry 200 packages each and small vans which can take 80 packages each. The cost of engaging each large van is ₹400 and each small van is ₹200. Not more than ₹3000 is to be spent daily on the job and the number of large vans cannot exceed the number of small vans. Formulate this problem as a LPP given that the objective is to minimize cost
The solution set of the inequation 2x + y > 5 is
Which of the following sets are convex?
The maximum value of Z = 4x + 3y subjected to the constraints 3x + 2y ≥ 160, 5x + 2y ≥ 200, x + 2y ≥ 80; x, y ≥ 0 is
Consider a LPP given by
Minimum Z = 6x + 10y
Subjected to x ≥ 6; y ≥ 2; 2x + y ≥ 10; x, y ≥ 0
Redundant constraints in this LPP are
The objective function Z = 4x + 3y can be maximised subjected to the constraints 3x + 4y ≤ 24, 8x + 6y ≤ 48, x ≤ 5, y ≤ 6; x, y ≥ 0
The optimum value of the objective function of LPP occurs at the center of the feasible region.
Choose the correct alternative:
The constraint that in a college there are more scholarship holders in FYJC class (X) than in SYJC class (Y) is given by
Choose the correct alternative:
How does a constraint, “A washing machine can hold up to 8 kilograms of cloths (X)” can be given?
State whether the following statement is True or False:
The half-plane represented by 3x + 4y ≥ 12 includes the point (4, 3)
By spending almost ₹ 250, Rakhi bought some kg grapes (x) and some dozens of bananas (y), then as a constraint this information can be expressed by ______
A doctor prescribed 2 types of vitamin tablets, T1 and T2 for Mr. Dhawan. The tablet T1 contains 400 units of vitamin and T2 contains 250 units of vitamin. If his requirement of vitamin is at least 4000 units, then the inequation for his requirement will be ______
Heramb requires at most 400 calories from his breakfast. Every morning he likes to take oats and milk. If each bowl of oats and a glass of milk provides him 80 calories and 50 calories respectively, then as a constraint this information can be expressed as ______
Ms. Mohana want to invest at least ₹ 55000 in Mutual funds and fixed deposits. Mathematically this information can be written as ______
Determine the maximum value of Z = 4x + 3y if the feasible region for an LPP is shown in figure
Determine the minimum value of Z = 3x + 2y (if any), if the feasible region for an LPP is shown in Figue.
Solve the following LPP graphically:
Maximise Z = 2x + 3y, subject to x + y ≤ 4, x ≥ 0, y ≥ 0
The common region determined by all the linear constraints of a LPP is called the ______ region.
