Advertisements
Advertisements
प्रश्न
A cylindrical tube of radius 12cm contains water to a depth of 20cm. A spherical ball of radius 9cm is dropped into the tube and thus level of water is raised by hcm. What is the value of h.
उत्तर
Given that radius of cylindrical tube (r1) = 12cm
Let height of cylindrical tube (h)
Volume of a cylinder = `pir_1^2h`
v1 = π(12)2 x h ............(1)
Given spherical ball radius (r2) = 9cm
Volume of sphere = `4/3pir_2^3`
`v_2=4/3xxpixx9^2` ..............(2)
Equating (1) and (2)
v1 = v2
`pi(12)^2xxh=4/3xxpixx9^3`
`h=(4/3xxpixx9^3)/(pi(12)^2)`
h = 6.75cm
Level of water raised in tube (h) = 6.75cm
APPEARS IN
संबंधित प्रश्न
A 16m deep well with diameter 3.5m is dug up and the earth from it is spread evenly to form a platform 27.5m by 7m. Find height of platform?
A well of diameter 2m is dug14m deep. The earth taken out of it is spread evenly all around it to form an embankment of height 40cm. Find width of the embankment?
Metal spheres each of radius 2cm are packed into a rectangular box of internal dimension 16cm x 8cm x 8cm when 16 spheres are packed the box is filled with preservative liquid. Find volume of this liquid?
A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 7 cm and the height of the cone is equal to its diameter. Find the volume of the solid. [Use`pi22/7`]
The radii of internal and external surfaces of a hollow spherical shell are 3 cm and 5 cm, respectively. It is melted and recast into a solid cylinder of diameter 14 cm. Find the height of the cylinder.
The diameter of a copper sphere is 18 cm. It is melted and drawn into a long wire of uniform cross-section. If the length of the wire is 108 m, find its diameter.
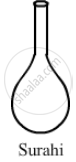
A surahi is a combination of
Water in a canal, 6 m wide and 1.5 m deep, is flowing with a speed of 10 km/hour. How much area will it irrigate in 30 mins; if 8 cm standing water is needed?
A cone of radius 8 cm and height 12 cm is divided into two parts by a plane through the mid-point of its axis parallel to its base. Find the ratio of the volumes of two parts.
The largest sphere is carved out of a solid cube of side 21 cm. Find the volume of the sphere.
