Advertisements
Advertisements
प्रश्न
A heap of wheat is in the form of a cone of diameter 9 m and height 3.5 m. Find its volume. How much canvas cloth is required to just cover the heap? (Use 𝜋 = 3.14).
Find the weight of a solid cone whose base is of diameter 14 cm and vertical height 51 cm, supposing the material of which it is made weighs 10 grams per cubic cm.
उत्तर १
Given diameter of cone 14cm
∴ Radius of cone=7cm
Height of cone =51cm
∴ Volume of cone=`1/3xxpir^2h`
`=1/3xx22/7xx7xx5xx51`
`= 2618 cm^3`
It is given that` 1cm^3` weight 10gm
`∴ 2618cm^3 weight (261xx10) gm `
i.e, 26.180kg.
उत्तर २
To find the weight of the cone we first need to find its volume.
The formula of the volume of a cone with base radius ‘r’ and vertical height ‘h’ is given as
Volume of cone = `1/3 pi r^2h`
Here, the diameter is given as 14 cm. From this we get the base radius as r = 7 m.
Substituting the values of r = 7 cm and h = 51 cm in the above equation and using `pi=22/7`
Volume = `((22)(7)(7)(51))/((3)(7))`
= (22) (7) (17)
= 2618
Hence the volume of the given cone with the specified dimensions is 2618 m3
Now, it is given that material of which the cone is made up of weighs 10 grams per cubic meter.
Hence the entire weight of the cone = (Volume of the cone) (10)
= (2618) (10)
= 26180 gram
Hence the weight of the cone is 26.18 kg
APPEARS IN
संबंधित प्रश्न
The radius of a cone is 5 cm and vertical height is 12 cm. Find the area of the curved surface.
The circumference of the base of a 10 m height conical tent is 44 metres. Calculate the length of canvas used in making the tent if width of canvas is 2 m. (Use it 𝜋= 22/7).
A solid cone of height 8 cm and base radius 6 cm is melted and recast into identical cones, each of height 2 cm and diameter 1 cm. Find the number of cones formed.
The internal and external diameter of a hollow hemispherical vessel are 21 cm and 28 cm respectively. Find :
- internal curved surface area,
- external curved surface area,
- total surface area,
- volume of material of the vessel.
The radii of the bases of two solid right circular cones of same height are r1 and r2 respectively. The cones are melted and recast into a solid sphere of radius R. Find the height of each cone in terms r1, r2 and R.
A buoy is made in the form of a hemisphere surmounted by a right circular cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 m and its volume is two-third the volume of hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two decimal places.
The internal and external diameters of a hollow hemi-spherical vessel are 21 cm and 28 cm respectively. Find: external curved surface area .
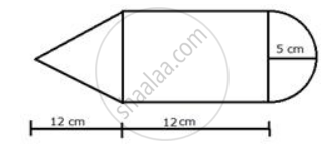
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.
A vessel in the form of an inverted cone is filled with water to the brim: Its height is 20 cm and the diameter is 16.8 cm. Two equal solid cones are dropped in it so that they are fully submerged. As a result, one-third of the water in the original cone overflows. What is the volume of each of the solid cones submerged?
The ratio of the radii of two right circular cones of the same height is 1 : 3. Find the ratio of their curved surface area when the height cone is 3 times the radius of the smaller cone.
