Advertisements
Advertisements
प्रश्न
A buoy is made in the form of a hemisphere surmounted by a right circular cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 m and its volume is two-third the volume of hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two decimal places.
उत्तर १
Radius of hemispherical part (r) = 3.5 m = `7/2`m
Therefore, Volume of hemisphere = `2/3pir^3`
= `2/3 xx 22/7 xx 7/2 xx 7/2 xx 7/2`
= `539/6`m3
Volume of conical part = `2/3 xx 539/6` m3 (2/3 of hemisphere)
Let height of the cone = h
Then ,
`1/3pir^2h = (2 xx 539)/(3 xx 6)`
⇒ `1/3 xx 22/7 xx 7/2 xx 7/2 xx h = (2 xx 539)/(3 xx 6)`
⇒ `h = (539 xx 2 xx 2 xx 7 xx 3)/(3 xx 6 xx 22 xx 7 xx 7)`
⇒ h = `14/3`m = 4`2/3`m = 4.67 m
Height of the cone = 4.67 m
Surface area of buoy = `2pir^2 + pirl`
But `l = sqrt(r^2 + h^2)`
`l = sqrt((7/2)^2 + (14/3)^2)`
= `sqrt(49/4 + 196/9) = sqrt(1225/36) = 35/6`m
Therefore , surface area =
= `(2 xx 22/7 xx 7/2 xx 7/2) + (22/7 xx 7/2 xx 35/6)`m2
= `77/1 + 385/6 = 847/6`
= 141.17 m2
उत्तर २
According to question.
`2/3` (Volume of hemisphere) = Volume of Cone
`2/3 ( 2/3 πr^3 ) = 1/3 πr^2h`
`4/9 (3.5)^3 = 1/3 (3.5)^2.h`
h = `(4 xx 3.5 xx 3.5 xx 3.5 xx 3)/(3.5 xx 3.5 xx 9)`
= `42.0/9 = 14/3 "m" = 4.67 "m"`
l = `sqrt (r^2 + h^2)`
= `sqrt((3.5)^2 + (4.67)^2)`
= `35/6` m
Now surface area of buoy = Surface area of right cone + Surface area of hemisphere
= πrl + 2πr2
= πr (l + 2r )

= `22/7 xx 3.5 (35/6 + 2 xx 3.5 )`
= 11 x (5.83 + 7)
= 11 x 12.83
= 141.13 sq.m.
APPEARS IN
संबंधित प्रश्न
A conical tent is 10 m high and the radius of its base is 24 m. Find
- slant height of the tent.
- cost of the canvas required to make the tent, if the cost of 1 m2 canvas is ₹ 70.
`["Assume "pi=22/7]`
A joker’s cap is in the form of right circular cone of base radius 7 cm and height 24 cm. Find the area of the sheet required to make 10 such caps.
`["Assume "pi=22/7]`
The area of the curved surface of a cone is 60 cm2. If the slant height of the cone be 8 cm, find the radius of the base?
The curved surface area of a cone is 4070 cm2 and its diameter is 70 cm. What is its slant height? (Use it 𝜋 = 22/7).
What length of tarpaulin 3 m wide will be required to make a conical tent of height 8 m and base radius 6 m? Assume that the extra length of material will be required for stitching margins and wastage in cutting is approximately 20 cm (Use it 𝜋 = 3.14)
The radius and height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the slant height and radius of the cone. (Use it 𝜋 = 3.14).
If the radius of the base of a cone is halved, keeping the height same, what is the ratio of the volume of the reduced cone to that of the original cone?
Find the volume of the largest right circular cone that can be fitted in a cube whose edge is 14 cm.
Find what length of canvas, 1.5 m in width, is required to make a conical tent 48 m in diameter and 7 m in height. Given that 10% of the canvas is used in folds and stitchings. Also, find the cost of the canvas at the rate of Rs. 24 per metre.
A solid metallic cone, with radius 6 cm and height 10 cm, is made of some heavy metal A. In order to reduce its weight, a conical hole is made in the cone as shown and it is completely filled with a lighter metal B. The conical hole has a diameter of 6 cm and depth 4 cm. Calculate the ratio of the volume of metal A to the volume of the metal B in the solid.
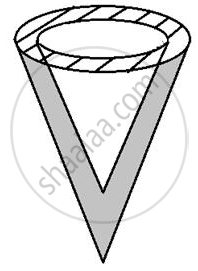
A cone of height 15 cm and diameter 7 cm is mounted on a hemisphere of same diameter. Determine the volume of the solid thus formed.
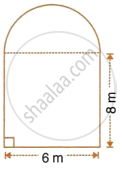
The cross-section of a railway tunnel is a rectangle 6 m broad and 8 m high surmounted by a semi-circle as shown in the figure. The tunnel is 35 m long. Find the cost of plastering the internal surface of the tunnel (excluding the floor) at the rate of Rs. 2.25 per m2.
The heights of two cones are in the ratio 1:3 and their base radii are in the ratio 3:1. Find the ratio of their volumes.
The radius and height of a cylinder, a cone and a sphere are same. Calculate the ratio of their volumes.
The surface area of a solid sphere is increased by 21% without changing its shape. Find the percentage increase in its: volume
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.
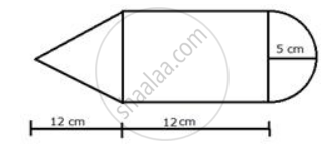
The radius and height of cone are in the ratio 3 : 4. If its volume is 301.44 cm3. What is its radius? What is its slant height? (Take π = 3.14)
A conical tent is accommodate to 11 persons each person must have 4 sq. metre of the space on the ground and 20 cubic metre of air to breath. Find the height of the cone.
The curved surface area of a right circular cone of height 15 cm and base diameter 16 cm is
