Question

A conical tent is 10 m high and the radius of its base is 24 m. Find

1. slant height of the tent.
2. cost of the canvas required to make the tent, if the cost of 1 m² canvas is ₹ 70.

`["Assume "pi=22/7]`

Answer 1

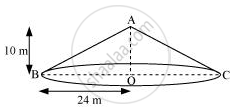
(i) Let ABC be a conical tent.

Height (h) of conical tent = 10 m

Radius (r) of conical tent = 24 m

Let the slant height of the tent be l.

In ΔABO,

AB² = AO² + BO²

l² = h² + r²

= (10 m)² + (24 m)²

= 676 m²

∴ l = 26 m

Therefore, the slant height of the tent is 26 m.

(ii) Curved surface area of the tent = πrl

= `(22/7xx24xx26)m^2`

= `13728/7  m^2`

Cost of 1 m² canvas = ₹ 70

`"Cost of "13728/7  m^2 " canvas"` = `₹ (13728/7xx70)`

= ₹ 137280

Therefore, the cost of the canvas required to make such a tent is ₹ 137280.