Advertisements
Advertisements
рдкреНрд░рд╢реНрди
What length of tarpaulin 3 m wide will be required to make a conical tent of height 8 m and base radius 6 m? Assume that the extra length of material will be required for stitching margins and wastage in cutting is approximately 20 cm (Use it ЁЭЬЛ = 3.14)
рдЙрддреНрддрд░
Given that,
Height of conical tent (h) =8m.
Radius of base of tent (r) = 6m
Slant height (l)`=sqrt(r^2+h^2)=sqrt(8^2+6^2)=sqrt100=10m`
CSA of conical tent = `pirl=(3.14xx6xx10)m^2`
`=188.4m^2`
Let the length of tarpaulin sheet require be L
As 200m will be wasted, So effective length will be (L-0.2m)
Breadth of tarpaulin = 3m
Area of sheet = CSA of sheet
`(Lxx0.2mxx3)m=188.40m^2 `
`⇒ L-0.2m=62.8m `
⇒ L=63m
Thus, the length of the tarpaulin sheet will be =163m .
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНтАНрди
Find the curved surface area of a cone, if its slant height is 60 cm and the radius of its base is 21 cm.
The radius and the height of a right circular cone are in the ratio 5 : 12. If its volume is 314 cubic meter, find the slant height and the radius (Use it ЁЭЬЛ = 3.14).
The radius and the height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the radius and slant height of the cone. (Take π = 3.14)
A heap of wheat is in the form of a cone of diameter 16.8 m and height 3.5 m. Find its volume. How much cloth is required to just cover the heap?
A solid metal sphere is cut through its center into 2 equal parts. If the diameter of the sphere is `3 1/2 cm`, find the total surface area of each part correct to two decimal places.
Total volume of three identical cones is the same as that of a bigger cone whose height is 9 cm and diameter 40 cm. Find the radius of the base of each smaller cone, if height of each is 108 cm
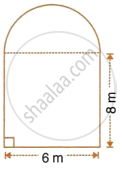
The cross-section of a railway tunnel is a rectangle 6 m broad and 8 m high surmounted by a semi-circle as shown in the figure. The tunnel is 35 m long. Find the cost of plastering the internal surface of the tunnel (excluding the floor) at the rate of Rs. 2.25 per m2.
Find the curved surface area of a cone whose height is 8 cm and base diameter is 12 cm .
Find the height of the cone whose base radius is 5 cm and volume is 75π cm3.
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the density of the material if its total weight is 1.7 kg
