Advertisements
Advertisements
प्रश्न
A hemispherical and a conical hole is scooped out of a.solid wooden cylinder. Find the volume of the remaining solid where the measurements are as follows:
The height of the solid cylinder is 7 cm, radius of each of hemisphere, cone and cylinder is 3 cm. Height of cone is 3 cm.
Give your answer correct to the nearest whole number.Taken`pi = 22/7`.

उत्तर
Required volume = Volume of cylinder – Volume of hemisphere – Volume of Cone ...(1)
For cone = Volume `= 1/3 pi r_1^2 h-1`
`= 1/3 xx pi xx(3)^2 xx 3`
`= 9 pi " cm"^3` ...(2)
For Hemisphere = Volume ` = 2/3 pi r _ 2^3 `
` = 2/3 xx pi xx (3)^3`
`= 18 pi " cm"^3 ` ...(3)
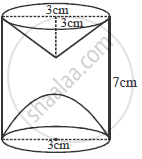
For cylinder = Volume `= pi r _3^2 h _3`
` = pi xx (3)^2 xx 7`
` = 63 pi` ...(4)
∴ Required volume = 63π - 18π - 9π {From (1), (2), (3) and (4)}
= 36 π
`= 36 xx 22/7 " cm"^3`
` = 113.14 " cm"^3`
` = 113 " cm"^3`
APPEARS IN
संबंधित प्रश्न
Find the surface area of a sphere of diameter 14 cm.
`["Assume "pi=22/7]`
Find the total surface area of a hemisphere of radius 10 cm. [Use π = 3.14]
Find the surface area of a sphere of diameter 3.5 cm .
The surface area of a sphere is 5544 `cm^2`, find its diameter.
The dome of a building is in the form of a hemisphere. Its radius is 63 dm. Find the cost of
painting it at the rate of Rs. 2 per sq. m.
Assuming the earth to be a sphere of radius 6370 km, how many square kilo metres is area
of the land, if three-fourth of the earth’s surface is covered by water?
A solid rectangular block of metal 49 cm by 44 cm by 18 cm is melted and formed into a solid sphere. Calculate the radius of the sphere.
The total area of a solid metallic sphere is 1256 cm2. It is melted and recast into solid right circular cones of radius 2.5 cm and height 8 cm. Calculate :
- the radius of the solid sphere.
- the number of cones recast. [Take π = 3.14]
Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r cm.
The total surface area of a hemisphere of radius r is
The largest sphere is cut off from a cube of side 6 cm. The volume of the sphere will be
The ratio between the volume of a sphere and volume of a circumscribing right circular cylinder is
If the radius of a solid hemisphere is 5 cm, then find its curved surface area and total surface area. ( π = 3.14 )
Find the volume of a sphere, if its surface area is 154 sq.cm.
Find the volume of the hollow sphere whose inner diameter is 8 cm and the thickness of the material of which it is made is 1 cm .
The volume of a sphere is 905 1/7 cm3, find its diameter.
There is a ratio 1: 4 between the surface area of two spheres, find the ratio between their radius.
A spherical cannon ball, 28 cm in diameter is melted and recast into a right circular conical mould, the base of which is 35 cm in diameter. Find the height of the cone, correct to one place of decimal.
How many spherical bullets can be made out of a solid cube of lead whose edge measures 44 cm, each bullet being 4 cm in diameter?
