Advertisements
Advertisements
प्रश्न
A piece of cloth costs Rs. 300. If the piece was 5 metres longer and each metre of cloth costs Rs. 2 less, the cost of the piece would have remained unchanged. How long is the original piece of cloth and what is the rate per metre?
उत्तर
The total cost of cloth piece = Rs. 300
Let the length of the piece of cloth in the beginning = x m
Then cost of 1 metre = Rs. `(300)/x`
In second case, length of cloth = (x + 5)
Cost of 1 metre = Rs. `(300)/(x + 5)`
According to the condition,
`(300)/x - (300)/(x + 5)` = 2
⇒ `300(1/x - 1/(x + 5))` = 2
⇒ `300((x + 5 - x)/(x(x + 5)))` = 2
⇒ `(300 xx 5)/(x(x + 5))` = 2
⇒ `(150 xx 5)/(x(x + 5))` = 1 ...(Dividing by 2)
750 = x2 + 5x
⇒ x2 + 5x - 750 = 0
⇒ x2 + 30x - 25x - 750 = 0
⇒ (x + 30) -25(x + 30) = 0
⇒ (x + 30)(x - 25) = 0
Either x + 30 = 0,
then x = -30
which is not possible being negative
or
x - 25 = 0,
then x = 25
∴ Length of cloth piece in the begining = 25 metres
and rate per metre = Rs. `(300)/(25)` = Rs. 12.
APPEARS IN
संबंधित प्रश्न
Solve the following quadratic equation for x:
`x^2+(a/(a+b)+(a+b)/a)x+1=0`
The difference of squares of two number is 88. If the larger number is 5 less than twice the smaller number, then find the two numbers.
The sum of two natural numbers is 15 and the sum of their reciprocals is `3/10`. Find the numbers.
Find the values of k for which the roots are real and equal in each of the following equation:
\[4 x^2 + px + 3 = 0\]
The value of c for which the equation ax2 + 2bx + c = 0 has equal roots is
Solve the following quadratic equation using formula method only
x2 - 6x + 4 = 0
The perimeter of the right angled triangle is 60cm. Its hypotenuse is 25cm. Find the area of the triangle.
Solve the following equation by factorization
5x2 – 8x – 4 = 0 when x∈Q
2x articles cost Rs. (5x + 54) and (x + 2) similar articles cost Rs. (10x – 4), find x.
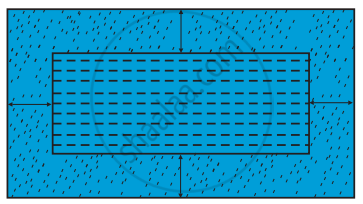
In the centre of a rectangular lawn of dimensions 50 m × 40 m, a rectangular pond has to be constructed so that the area of the grass surrounding the pond would be 1184 m2 [see figure]. Find the length and breadth of the pond.