Question

A semi-circular sheet of metal of diameter 28 cm is bent to form an open conical cup. Find the capacity of the cup.

Answer 1

Given, diameter of a semi-circular sheet = 28 cm

∴ Radius of a semi-circular sheet, r = `28/2` = 14 cm

Since, a semi-circular sheet of metal is bent to form an open conical cup.

Let the radius of a conical cup be R.

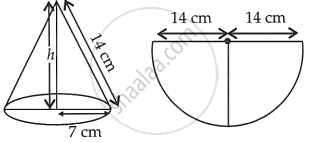
∴ Circumference base of cone = Circumference of semi-circle

2πR = πr

⇒ 2πR = π × 14

⇒ R = 7 cm

Now, `h = sqrt(l^2 - R^2)`  ...[∵ l² = h² + R²] 

= `sqrt(14^2 - 7^2)` 

= `sqrt(196 - 49)`

= `sqrt(147)`

= 12.1243 cm

Volume (capacity) of conical cup = `1/3 piR^2h`

= `1/3 xx 22/7 xx 7 xx 7 xx 12.1243`

= 622.38 cm³

Hence, the capacity of an open conical cup is 622.38 cm³.