Advertisements
Advertisements
प्रश्न
A swimming pool is 50 m long and 15 m wide. Its shallow and deep ends are 1.5 m and 4.5 m respectively. If the bottom of the pool slopes uniformly, find the amount of water in kilolitres required to fill the pool (1 m3 = 1000 liters).
उत्तर
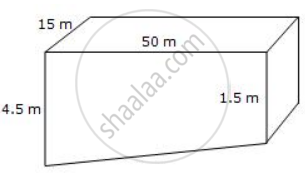
Area of cross section = Area of trapezium
= `(1)/(2) xx ("sum of parallel sides") xx "height"`
= `(1)/(2) xx (1.5 + 4.5) xx 50`
= `(1)/(2) xx 6 xx 50`
= 150m2
∴ Volume of the pool
= area of cross section x height
= 150 x 15
= 2250m3 ...(∵ 1m3 = 1kilolitres)
∴ Volume of the pool is 2250kilolitres.
APPEARS IN
संबंधित प्रश्न
A swimming pool is 40 m long and 15 m wide. Its shallow and deep ends are 1.5 m and 3 m deep respectively. If the bottom of the pool slopes uniformly, find the amount of water in liters required to fill the pool.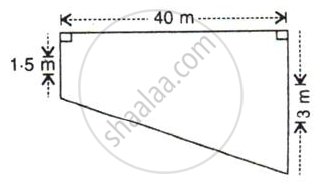
A swimming pool is 18 m long and 8 m wide. Its deep and shallow ends are 2 m and 1.2 m respectively. Find the capacity of the pool, assuming that the bottom of the pool slopes uniformly.
A rectangular cardboard sheet has length 32 cm and breadth 26 cm. Squares each of side 3 cm, are cut from the corners of the sheet and the sides are folded to make a rectangular container. Find the capacity of the container formed.
A school auditorium is 40 m long, 30 m broad and 12 m high. If each student requires 1.2 m2 of the floor area; find the maximum number of students that can be accommodated in this auditorium. Also, find the volume of air available in the auditorium, for each student.
The internal dimensions of a rectangular box are 12 cm x `x` cm x 9 cm. If the length of the longest rod that can be placed in this box is 17 cm; find `x`.
A rectangular field is 112 m long and 62 m broad. A cubical tank of edge 6 m is dug at each of the four corners of the field and the earth so removed is evenly spread on the remaining field. Find the rise in level.
Find the length of 22 kg copper wire of diameter 0.8 cm, if the weight of 1 cm3 copper is 4.2 g.
The cross section of a piece of metal 2 m in length is shown. Calculate the area of cross section.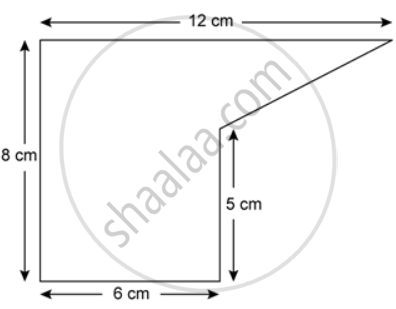
ABCDE is the end view of a factory shed which is 50 m long. The roofing of the shed consists of asbestos sheets as shown in the figure. The two ends of the shed are completely closed by brick walls.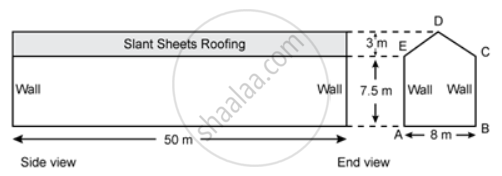
Calculate the total volume content of the shed.
The cross section of a swimming pool is a trapezium whose shallow and deep ends are 1 m and 3 m respectively. If the length of the pool is 50 m and its width is 1.5 m, calculate the volume of water it holds.
