Advertisements
Advertisements
प्रश्न
A triangle has sides 35 cm, 54 cm and 61 cm long. Find its area. Also, find the smallest of its altitudes ?
उत्तर
The sides of a triangle are a = 35 cm, b = 54 cm and c = 61 cm
Now, perimeter a + b + c = 25
`⇒S= 1/2(35+54+61)`
⇒s= 75cm
By using heron’s formula
∴Area of triangle =`sqrt(s(s-a)(s-a)(s-c))`
`=sqrt(75(75-35)(75-54)(75-61))`
`sqrt(75(40)(21)(14))=93914cm^2`
∴ The altitude will be a smallest when the side corresponding to it is longest Here, longest side is 61 cm
∴ area altitude will be a smallest when the side corresponding to is longest here' longest side is 61 cm
∴area of Δ le = `1/2xxbxxh= 1/2xx base xx height `
`∴1/2xxhxx61= 939.14`
`⇒= (939.14xx2)/61= 30.79 cm`
𝐻𝑒𝑛𝑐𝑒 𝑡ℎ𝑒 𝑙𝑒𝑛𝑔𝑡ℎ 𝑜𝑓 𝑡ℎ𝑒 𝑠𝑚𝑎𝑙𝑙𝑒𝑠𝑡 𝑎𝑙𝑡𝑖𝑡𝑢𝑑𝑒 𝑖𝑠 30.79 𝑐𝑚
APPEARS IN
संबंधित प्रश्न
Find the area of an isosceles triangle having the base x cm and one side y cm.
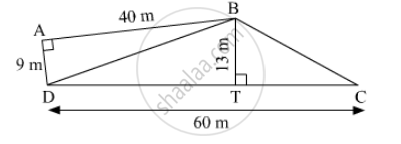
Some measures are given in the adjacent figure, find the area of ☐ABCD.
Determine whether the sets of points are collinear?
`(-1/2, 3), (-5, 6) and (-8, 8)`
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
Find the area of triangle FED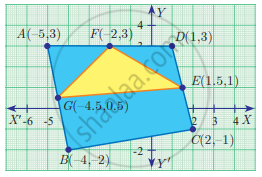
If the side of a rhombus is 10 cm and one diagonal is 16 cm, the area of the rhombus is 96 cm2.
The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 13 m, 14 m and 15 m. The advertisements yield an earning of Rs 2000 per m2 a year. A company hired one of its walls for 6 months. How much rent did it pay?
A field in the form of a parallelogram has sides 60 m and 40 m and one of its diagonals is 80 m long. Find the area of the parallelogram.
A rhombus shaped sheet with perimeter 40 cm and one diagonal 12 cm, is painted on both sides at the rate of Rs 5 per m2. Find the cost of painting.
The perimeter of a triangle is 50 cm. One side of a triangle is 4 cm longer than the smaller side and the third side is 6 cm less than twice the smaller side. Find the area of the triangle.
