Advertisements
Advertisements
प्रश्न
A vehicle is moving on a circular track whose surface is inclined towards the horizon at an angle of 10°. The maximum velocity with which it can move safely is 36 km / hr. Calculate the length of the circular track. [π = 3.142]
उत्तर
Given, angle of banking, θ = 10°
Optimum speed, V0 = 36 km/hr = 36 x `5/18` m/s.
Or, V0 = 10 m/s
Let R be the radius of the circular track
We have,
V0 = `sqrt( gRtanθ )`
⇒ `V_0^2` = gRtanθ
⇒ R = `V_0^2/[ g tanθ ]`
= `(10m/s)^2/[(9.8m/s^2) xx tan 10^0]`
= `[100m]/[ 9.8 xx 0.1763 ]`
⇒ R = 57.88 m
∴ Length of the circular track = 2πR = 2 x 3.142 x 57.88 = 363.72m.
APPEARS IN
संबंधित प्रश्न
Read the statement below carefully and state, with reason, if it is true or false:
The velocity vector of a particle at a point is always along the tangent to the path of the particle at that point.
Read the statement below carefully and state, with reason, if it is true or false:
The acceleration vector of a particle in uniform circular motion averaged over one cycle is a null vector.
Is it possible to have an accelerated motion with a constant speed? Explain
Draw a neat labelled diagram for a particle moving in a circular path with a constant speed. In you diagram show the direction of velocity at any instant.
| A small pebble tied at one end of a string is placed near the periphery of a circular disc, at the centre of which the other end of the string is tied to a peg. The disc is rotating about an axis passing through its centre. |
- What will be your observation when you are standing outside the disc? Explain.
- What will be your observation when you are standing at the centre of the disc? Explain.
A uniform metre rule of mass 100g is balanced on a fulcrum at mark 40cm by suspending an unknown mass m at the mark 20cm.
To which side the rule will tilt if the mass m is moved to the mark 10cm ?
Answer the following question.
Show that its time period is given by, 2π`sqrt((l cos theta)/("g"))` where l is the length of the string, θ is the angle that the string makes with the vertical, and g is the acceleration due to gravity.
What is meant by uniform circular motion? Give two examples of uniform circular motion.
The angle subtended by the vector A = `5hat"i" + 3hat"j" + 12hat"k"` with the X-axis is ______.
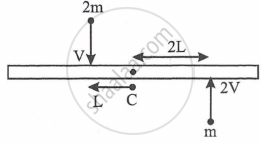
A uniform rod of length '6L' and mass '8 m' is pivoted at its centre 'C'. Two masses 'm' and ' 2m' with speed 2v, v as shown strikes the rod and stick to the rod. Initially the rod is at rest. Due to impact, if it rotates with angular velocity 'w1' then 'w' will be ________.
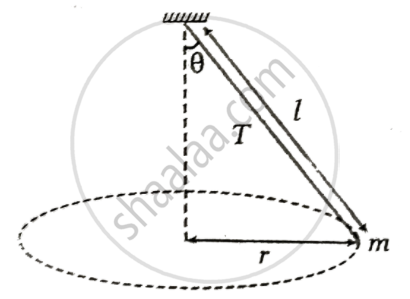
A string of length 'l' fixed at one end carries a mass 'm' at the other end. The string makes `3/pi` revolutions/second around the vertical axis through the fixed end as shown in figure. The tension 'T' in the string is ______.
Two particles P and Q are moving in concentric circles of rarui rp and rQ respectively. If their period of revolutions are in ratio 2 : 3, then ratio of their centripetal acceleration is ____________.
The angular speed of the minute hand of a clock in degrees per second is ______.
A body moves in a uniform circular motion ______.
Statement A: Uniform circular motion is a case of accelerated motion
Statement B: In the third equation of motion we do not have the term time
A body is said to be in nonuniform motion if it travels ______.
A flywheel at rest is to reach an angular velocity of 24 rad/s in 8 second with constant angular acceleration. The total angle turned through during this interval is ______.
If a body is moving in a circle of radius r with a constant speed v, its angular velocity is ______.
A cyclist starts from centre O of a circular park of radius 1 km and moves along the path OPRQO as shown figure. If he maintains constant speed of 10 ms–1, what is his acceleration at point R in magnitude and direction?

Earth can be thought of as a sphere of radius 6400 km. Any object (or a person) is performing circular motion around the axis of earth due to earth’s rotation (period 1 day). What is acceleration of object on the surface of the earth (at equator) towards its centre? what is it at latitude θ? How does these accelerations compare with g = 9.8 m/s2?
A small bead of mass m can move on a smooth circular wire (radius R) under the action of a force F = `"Km"/"r"^2` directed (r = position of bead r from P and K = constant) towards a point P within the circle at a distance R/2 from the centre. The minimum velocity should be ______ m/s of bead at the point of the wire nearest the centre of force (P) so that bead will complete the circle. (Take `"k"/(3"R")` = 8 unit)

A wet open umbrella is held upright and is rotated about the handle at a uniform rate of 21 revolutions in 44 s. If the rim of the umbrella is a circle of 1 metre in diameter and the height of the rim above the floor is 1.5 m, the drops of water spun off the rim and hit the floor at a horizontal ______ m from the umbrella.
A horizontal circular platform of mass M is rotating at angular velocity ω about a vertical axis passing through its centre. A boy of mass m is standing at the edge of the platform. If the boy comes to the centre of the platform, then the new angular velocity becomes ______.
Two bodies of masses 10 kg and 5 kg moving in concentric orbits of radii R and r such that their periods are the same. Then the ratio between their centripetal accelerations is ______.
A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω. Another disc of same dimensions, but of mass `1/4`M is placed gently on the first disc co-axially. The. angular velocity of the system is ______.
A wheel is Subjected to uniform angular acceleration about its axis. Initially. its angular velocity is zero. In the first 2 s, it rotates through an angle θ1, in the next 2 s, it rotates through an angle θ2. The ratio of `theta_2/theta_1` is ______.
Why is uniform circular motion said to be accelerated?
