Advertisements
Advertisements
प्रश्न
A wooden toy was made by scooping out a hemisphere of same radius from each end of a solid cylinder. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the volume of wood in the toy. `[\text{Use}pi 22/7]`
उत्तर
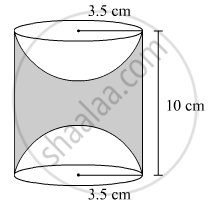
Height of the cylinder, h = 10 cm
Radius of the cylinder = Radius of each hemisphere = r = 3.5 cm
Volume of wood in the toy = Volume of the cylinder − 2 × Volume of each hemisphere
`=pir^2h-2xx2/3pir^2`
`=22/7xx(3.5cm)^2xx10cm-4/3xx22/7xx(3.5cm)^3`
`=385cm^3-539/3cm^3`
`=616/3cm^3`
`=205.33cm^3`(Approx)
Thus, the volume of the wood in the toy is approximately 205.33 cm3.
APPEARS IN
संबंधित प्रश्न
The diameter and length of a roller is 120 cm and 84 cm respectively. To level the ground, 200 rotations of the roller are required. Find the expenditure to level the ground at the rate of Rs. 10 per sq.m.
Find the mass of a 3.5 m long lead pipe, if the external diameter of the pipe is 2.4 cm, thickness of the metal is 2 mm and the mass of 1 cm3 of lead is 11.4 grams.
A cylindrical vessel 32 cm high and 18 cm as the radius of the base, is filled with sand. This bucket is emptied on the ground and a conical heap of sand is formed. If the height of the conical heap is 24 cm, the radius of its base is
The volume of a cube is 729 cm3. Find its surface area.
The interior of a building is in the form of a right circular cylinder of diameter 4.2 m and height 4 m surmounted by a cone of same diameter.
The height of the cone is 2.8 m. Find the outer surface area of the building.
The volumes of two spheres are in the ratio 64 : 27. The ratio of their surface areas is ______.
The surface area of a sphere is 154 cm2. The volume of the sphere is
A circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 40 m, the total area of canvas required is
The radius of the base and the height of a solid right circular cylinder are in the ratio 2 : 3 and its volume is 1617 cm3. Find the total surface area of the cylinder.

In Figure 3, a decorative block is shown which is made of two solids, a cube, and a hemisphere. The base of the block is a cube with an edge 6 cm and the hemisphere fixed on the top has a diameter of 4⋅2 cm. Find
(a) the total surface area of the block.
(b) the volume of the block formed. `("Take" pi = 22/7)`
