Advertisements
Advertisements
प्रश्न
ABC is a triangle whose vertices are A(1, –1), B(0, 4) and C(– 6, 4). D is the midpoint of BC. Find the coordinates of D.
उत्तर
D is the mid-point of BC.
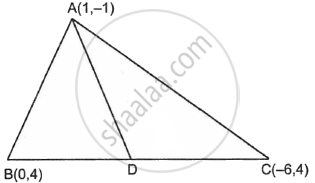
∴ Co-ordinates of D are `((0 - 6)/2, (4 + 4)/2)`
i.e., (–3, 4)
APPEARS IN
संबंधित प्रश्न
Find the mid-point of the line segment joining the points:
(5, –3) and (–1, 7)
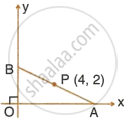
In the given figure, P(4, 2) is mid-point of line segment AB. Find the co-ordinates of A and B.
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
Find the coordinates of point P if P divides the line segment joining the points A(–1, 7) and B(4, –3) in the ratio 2 : 3.
Write the co-ordinates of the point of intersection of graphs of
equations x = 2 and y = -3.
A lies on the x - axis amd B lies on the y -axis . The midpoint of the line segment AB is (4 , -3). Find the coordinates of A and B .
P , Q and R are collinear points such that PQ = QR . IF the coordinates of P , Q and R are (-5 , x) , (y , 7) , (1 , -3) respectively, find the values of x and y.
The centre of a circle is (−4, 2). If one end of the diameter of the circle is (−3, 7) then find the other end
The coordinates of the point C dividing the line segment joining the points P(2, 4) and Q(5, 7) internally in the ratio 2 : 1 is
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
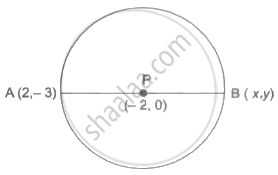
Given: A`square` and P`square`. Let B (x, y)
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
`square = (square + x)/square`
⇒ `square = square` + x
⇒ x = `square - square`
⇒ x = – 6
and `square = (square + y)/2`
⇒ `square` + y = 0
⇒ y = 3
Hence coordinates of B is (– 6, 3).
