Advertisements
Advertisements
प्रश्न
According to Stefan’s law of radiation, a black body radiates energy σT4 from its unit surface area every second where T is the surface temperature of the black body and σ = 5.67 × 10–8 W/m2K4 is known as Stefan’s constant. A nuclear weapon may be thought of as a ball of radius 0.5 m. When detonated, it reaches temperature of 106 K and can be treated as a black body.
- Estimate the power it radiates.
- If surrounding has water at 30°C, how much water can 10% of the energy produced evaporate in 1s? [Sw = 4186.0 J/kg K and Lv = 22.6 × 105 J/kg]
- If all this energy U is in the form of radiation, corresponding momentum is p = U/c. How much momentum per unit time does it impart on unit area at a distance of 1 km?
उत्तर
Given, σ = 5.67 × 10–8 W/m2 kg
Radius = R = 0.5 m, T = 106 K
a. Power radiated by Stefan's law
P = σAT4 = (4πR2)T4
= 5.67 × 10–4 × 4 × (3.14) × (0.5)2 × (106)4
= 1.78 × 1017 J/s
= 1.8 × 1017 J/s
b. Energy available per second, U = 1.8 × 1017 J/s = 18 × 1016 J/s
Actual energy required to evaporate water = 10% of 1.8 × 1017 J/s
= 1.8 × 1016 J/s
Energy used per second to raise the temperature of m kg of water 30°C to 100°C and then into vapour at 100°C
= msw Δθ + mLv
= m × 4186 × (100 – 30) + m × 22.6 × 105
= 2.93 × 105 m + 22.6 × 105
m = 25.53 × 105 m J/s
As per the question, 25.53 × 105 m = 1.8 × 1016
or m = `(1.8 xx 10^16)/(25.33 xx 10^5) = 7.0 xx 10^9` kg
c. Momentum per unit time,
p = `U/c = U/c`
= `(1.8 xx 10^17)/(3 xx 10^8)`
= `6 xx 10^8` kg-m/s2 ......`[(P = "momentum"),(V = "energy"),(C = "velocity of light")]`
Momentum per unit time per unit
Area p = `P/(4piR^2)`
= `(6 xx 10^8)/(4 xx 3.14 xx (10^3)^2`
⇒ d = 47.7 N/m2 .......[4πR2 = Surface area]
APPEARS IN
संबंधित प्रश्न
Explain why a brass tumbler feels much colder than a wooden tray on a chilly day
Two identical rectangular strips, one of copper and the other of steel, are riveted together to form a bimetallic strip (acopper> asteel). On heating, this strip will
A steel frame (K = 45 W m−1°C−1) of total length 60 cm and cross sectional area 0.20 cm2, forms three sides of a square. The free ends are maintained at 20°C and 40°C. Find the rate of heat flow through a cross section of the frame.
A cubical box of volume 216 cm3 is made up of 0.1 cm thick wood. The inside is heated electrically by a 100 W heater. It is found that the temperature difference between the inside and the outside surface is 5°C in steady state. Assuming that the entire electrical energy spent appears as heat, find the thermal conductivity of the material of the box.
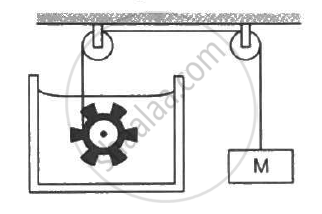
Following Figure shows water in a container having 2.0 mm thick walls made of a material of thermal conductivity 0.50 W m−1°C−1. The container is kept in a melting-ice bath at 0°C. The total surface area in contact with water is 0.05 m2. A wheel is clamped inside the water and is coupled to a block of mass M as shown in the figure. As the block goes down, the wheel rotates. It is found that after some time a steady state is reached in which the block goes down with a constant speed of 10 cm s−1 and the temperature of the water remains constant at 1.0°C. Find the mass M of the block. Assume that the heat flows out of the water only through the walls in contact. Take g = 10 m s−2.
A semicircular rod is joined at its end to a straight rod of the same material and the same cross-sectional area. The straight rod forms a diameter of the other rod. The junctions are maintained at different temperatures. Find the ratio of the heat transferred through a cross section of the semicircular rod to the heat transferred through a cross section of the straight rod in a given time.
Steam at 120°C is continuously passed through a 50 cm long rubber tube of inner and outer radii 1.0 cm and 1.2 cm. The room temperature is 30°C. Calculate the rate of heat flow through the walls of the tube. Thermal conductivity of rubber = 0.15 J s−1 m−1°C−1.
A room has a window fitted with a single 1.0 m × 2.0 m glass of thickness 2 mm. (a) Calculate the rate of heat flow through the closed window when the temperature inside the room is 32°C and the outside is 40°C. (b) The glass is now replaced by two glasspanes, each having a thickness of 1 mm and separated by a distance of 1 mm. Calculate the rate of heat flow under the same conditions of temperature. Thermal conductivity of window glass = 1.0 J s−1 m−1°C−1 and that of air = 0.025 m-1°C-1 .
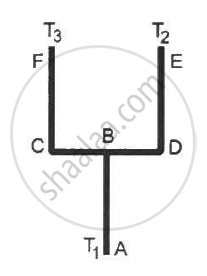
Four identical rods AB, CD, CF and DE are joined as shown in following figure . The length, cross-sectional area and thermal conductivity of each rod are l, A and K respectively. The ends A, E and F are maintained at temperature T1, T2 and T3 respectively. Assuming no loss of heat to the atmosphere, find the temperature at B.
Following figure shows two adiabatic vessels, each containing a mass m of water at different temperatures. The ends of a metal rod of length L, area of cross section A and thermal conductivity K, are inserted in the water as shown in the figure. Find the time taken for the difference between the temperatures in the vessels to become half of the original value. The specific heat capacity of water is s. Neglect the heat capacity of the rod and the container and any loss of heat to the atmosphere.

