Advertisements
Advertisements
प्रश्न
AD is a diameter of a circle and AB is a chord If AD = 30 cm and AB = 24 cm then the distance of AB from the centre of the circle is
पर्याय
10 cm
9 cm
8 cm
6 cm
उत्तर
9 cm
Explanation;
Hint: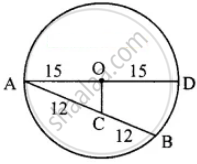
In ΔAOC,
AO = 15 cm
AC = `1/2` AB
= `1/2 xx 24`
= 12 cm
In ΔAOC,
OC2 = AO2 – AC2
= 152 – 122
= 225 – 144
= 81
OC = `sqrt(81)`
= 9 cm
APPEARS IN
संबंधित प्रश्न
In fig., circles C(O, r) and C(O’, r/2) touch internally at a point A and AB is a chord of the circle C (O, r) intersecting C(O’, r/2) at C, Prove that AC = CB.
Fill in the blanks:
A point, whose distance from the centre of a circle is greater than its radius lies in __________ of the circle. (exterior/ interior)
In the given figure, a circle with center O, is inscribed in a quadrilateral ABCD such that it touches the side BC, AB, AD and CD at points P, Q, R and S respectively. If AB = 29cm, AD = 23cm, ∠B = 90° and DS=5cm then find the radius of the circle.

If O is the centre of a circle of radius r and AB is a chord of the circle at a distance r/2 from O, then ∠BAO =
In the given figure, if ∠ABC = 45°, then ∠AOC =
Construct a triangle ABC with AB = 4.2 cm, BC = 6 cm and AC = 5cm. Construct the circumcircle of the triangle drawn.
Construct a triangle ABC with AB = 5 cm, ∠B = 60° and BC = 6. 4 cm. Draw the incircle of the triangle ABC.
Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord
In the adjoining figure, Δ ABC is circumscribing a circle. Then, the length of BC is ______

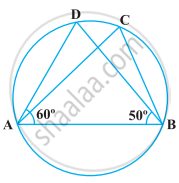
In the following figure, if ∠DAB = 60º, ∠ABD = 50º, then ∠ACB is equal to ______.