Advertisements
Advertisements
प्रश्न
If O is the centre of a circle of radius r and AB is a chord of the circle at a distance r/2 from O, then ∠BAO =
पर्याय
60°
45°
30°
15°
Advertisements
उत्तर
We will associate the given information in the following figure.
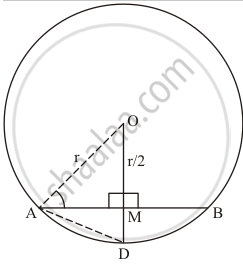
Since AO = r (radius of circle)
AM = `r/2` (given)
Extended OM to D where MD = `r/2`
Consider the triangles AOM and triangle AMD
OM = MD
`angleAMO = angle AMD` = 90°
AM = AM (common Sides
So by SSS property
Δ AMO ≅ Δ DM
So AD = AO = r and OD=OM+MD=r
Hence ΔAOD is equilateral triangle
So `angle OAD` = 60°
We know that in equilateral triangle altitudes divide the vertex angles
Therefore `angleOAM = (angleOAD)/2`
`=60/2`
= 30°
APPEARS IN
संबंधित प्रश्न
Two circles touch each other externally at P. AB is a common tangent to the circles touching them at A and B. The value of ∠ L APB is
(A) 30°
(B) 45°
(C) 60°
(D) 90°
A chord of a circle of radius 10 em subtends a right angle at its centre. The length of the chord (in em) is
`(A) 5sqrt 2`
`(B) 10 sqrt2`
`(C)5/sqrt2`
`(D) 10sqrt 3`
Prove that in two concentric circles, the chord of the larger circle which touches the smaller circle, is bisected at the point of contact.
In fig., O is the centre of the circle, PA and PB are tangent segments. Show that the quadrilateral AOBP is cyclic.
In the given figure, O is the centre of a circle. PT and PQ are tangents to the circle from an external point P. If ∠TPQ = 70°, find ∠TRQ.
Use the figure given below to fill in the blank:
If the length of RS is 5 cm, the length of PQ = _______
A chord is 12 cm away from the centre of the circle of radius 15 cm. Find the length of the chord
Circles with centres A, B and C touch each other externally. If AB = 3 cm, BC = 3 cm, CA = 4 cm, then find the radii of each circle.
A line through the point of contact and passing through centre of the circle is known as ______
If a number of circles pass through the endpoints P and Q of a line segment PQ, then their centres lie on the perpendicular bisector of PQ.
