Advertisements
Advertisements
प्रश्न
An area is paved with square tiles of a certain size and the number required is 128. If the tiles had been 2 cm smaller each way, 200 tiles would have been needed to pave the same area. Find the size of the larger tiles.
Advertisements
उत्तर
Let the size of the larger tiles be x cm.
Area of larger tiles = x2 cm2
Number of larger tiles required to pave an area is 128.
So, the area needed to be paved = 128x2 cm2 ...(1)
Size of smaller tiles = (x – 2) cm
Area of smaller tiles = (x – 2)2 cm2
Number of larger tiles required to pave an area is 200.
So, the area needed to be paved = 200(x – 2)2 cm2 ...(2)
Therefore, from (1) and (2), we have:
128x2 = 200(x – 2)2
128x2 = 200x2 + 800 – 800x
72x2 – 800x + 800 = 0
9x2 – 100x + 100 = 0
9x2 – 90x – 10x + 100 = 0
9x(x – 10) – 10(x – 10) = 0
(x – 10)(9x – 10) = 0
`x = 10,10/9`
If `x = 10/9`,
Then `x - 2 = 10/9 - 2`
= `(10 - 18)/9`
= `(-8)/9`
Which is not possible.
Hence, the size of the larger tiles is 10 cm.
संबंधित प्रश्न
The sides of a right-angled triangle containing the right angle are 4x cm and (2x – 1) cm. If the area of the triangle is 30 cm2; calculate the lengths of its sides.
The hypotenuse of a right-angled triangle is 26 cm and the sum of other two sides is 34 cm. Find the lengths of its sides.
The hypotenuse of a right-angled triangle exceeds one side by 1 cm and the other side by 18 cm; find the lengths of the sides of the triangle.
The diagonal of a rectangle is 60 m more than its shorter side and the larger side is 30 m more than the shorter side. Find the sides of the rectangle.
A footpath of uniform width runs round the inside of a rectangular field 32 m long and 24 m wide. If the path occupies 208 m2, find the width of the footpath.
The dimensions of a rectangular field are 50 m and 40 m. A flower bed is prepared inside this field leaving a gravel path of uniform width all around the flower bed. The total cost of laying the flower bed and gravelling the path at Rs. 30 and Rs. 20 per square metre, respectively, is Rs. 52,000. Find the width of the gravel path.
A farmer has 70 m of fencing, with which he encloses three sides of a rectangular sheep pen; the fourth side being a wall. If the area of the pen is 600 sq. m, find the length of its shorter side.
The area of a big rectangular room is 300 m2. If the length were decreased by 5 m and the breadth increased by 5 m; the area would be unaltered. Find the length of the room.
In the given figure, the value of x is ______.
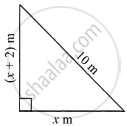
The area of the given rectangle is 60 sq. m and its longer side is 10 m, the perimeter of the rectangle is ______.
