Advertisements
Advertisements
प्रश्न
Consider a circular current-carrying loop of radius R in the x-y plane with centre at origin. Consider the line intergral
`ℑ(L ) = |int_(-L)^L B.dl|` taken along z-axis.
- Show that ℑ(L) monotonically increases with L.
- Use an appropriate Amperian loop to show that ℑ(∞) = µ0I, where I is the current in the wire.
- Verify directly the above result.
- Suppose we replace the circular coil by a square coil of sides R carrying the same current I. What can you say about ℑ(L) and ℑ(∞)?
Advertisements
उत्तर
Magnetic field due to a circular current-carrying loop lying in the xy-plane acts along z-axis as shown in figure.
a. B(z) points in the same direction of z-axis and hence ℑ(L) is monotonical function of L as B and dl are long in the same direction.
So B.dl = Bdl cos θ = Bdl cos θ° = Bdl
∴ ℑ(L) is monotonically increasing function of L.
b. Now consider as Amperean loop around the circular coil of such a large radius that L → `oo`. Since this loop encloses a current I, Now using Ampere's law
`ℑ(oo) = oint_(-oo)^(+oo) vec(B) * vec(dl) = mu_0I`
c. The magnetic field at the axis (z-axis) of circular coil is given by `B_z = (mu_0IR^2)/(2(z^2 + R^2)^(3/2)`
Now integrating
`int_(-oo)^(+oo) B_z dz = int_(-oo)^(+oo) (mu_0IR^2)/(2(z^2 + R^2)^(3/2)) dz`
Let z = R tan θ so that dz = R sec2 θ dθ
And `(z^2 + R^2)^(3/2) = (R^2 tan^2 theta + R^2)^(3/2)`
= `R^3 sec^3θ (as 1 + tan^2θ = sec^2θ)`
Thus `int_(-oo)^(+oo) B_zdz = (mu_0I)/2 int_(- pi/2)^(+ pi/2) (R^2(R sec^2 θ dθ))/(R^3 sec^3θ)`
= `(mu_0I)/2 int_(- pi/2)^(+ pi/2) cos θ dθ = mu_0I`
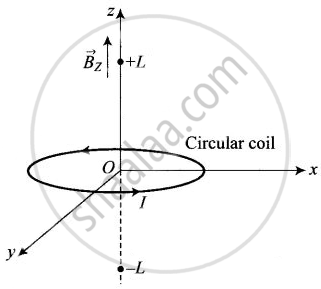
d. As we know `(B_z)_("square") < (B_z)_("circular coil")`
For the same current and side of the square equal to radius of the coil
`ℑ(oo)_("square") < ℑ(oo)_("circular coil")`
By using the same argument as we done in case (b), it can be shown that `ℑ(oo)_("square") < ℑ(oo)_("circular coil")`
APPEARS IN
संबंधित प्रश्न
Use Biot-Savart law to derive the expression for the magnetic field on the axis of a current carrying circular loop of radius R.
Draw the magnetic field lines due to a circular wire carrying current I.
Two identical circular coils, P and Q each of radius R, carrying currents 1 A and √3A respectively, are placed concentrically and perpendicular to each other lying in the XY and YZ planes. Find the magnitude and direction of the net magnetic field at the centre of the coils.
At a place, the horizontal component of earth's magnetic field is B and angle of dip is 60°. What is the value of horizontal component of the earth's magnetic field at equator?
Derive the expression for the torque on a rectangular current carrying loop suspended in a uniform magnetic field.
A current-carrying, straight wire is kept along the axis of a circular loop carrying a current. This straight wire
A circular loop is kept in that vertical plane which contains the north-south direction. It carries a current that is towards north at the topmost point. Let A be a point on the axis of the circle to the east of it and B a point on this axis to the west of it. The magnetic field due to the loop
Consider the situation shown in figure. The straight wire is fixed but the loop can move under magnetic force. The loop will
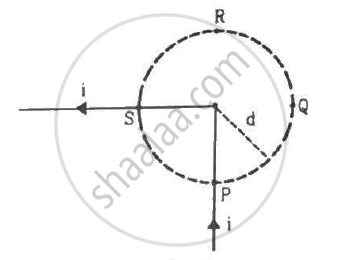
Figure shows a long wire bent at the middle to form a right angle. Show that the magnitudes of the magnetic fields at the point P, Q, R and S are equal and find this magnitude.
A circular loop of radius 20 cm carries a current of 10 A. An electron crosses the plane of the loop with a speed of 2.0 × 106 m s−1. The direction of motion makes an angle of 30° with the axis of the circle and passes through its centre. Find the magnitude of the magnetic force on the electron at the instant it crosses the plane.
A circular loop of radius R carries a current I. Another circular loop of radius r(<<R) carries a current i and is placed at the centre of the larger loop. The planes of the two circles are at right angle to each other. Find the torque acting on the smaller loop.
A circular loop of radius r carrying a current i is held at the centre of another circular loop of radius R(>>r) carrying a current I. The plane of the smaller loop makes an angle of 30° with that of the larger loop. If the smaller loop is held fixed in this position by applying a single force at a point on its periphery, what would be the minimum magnitude of this force?
Find the magnetic field B due to a semicircular wire of radius 10.0 cm carrying a current of 5.0 A at its centre of curvature.
A charge of 3.14 × 10−6 C is distributed uniformly over a circular ring of radius 20.0 cm. The ring rotates about its axis with an angular velocity of 60.0 rad s−1. Find the ratio of the electric field to the magnetic field at a point on the axis at a distance of 5.00 cm from the centre.
Which of these equations is the correct expression for force on a charge in magnetic field?
The magnitude of the magnetic field due to a circular coil of radius R carrying a current I at an axial distance x from the centre is ______.
The magnetic field at a distance r from a long wire carrying current I is 0.4 tesla. The magnetic field at a distance 2 r is ______.
A charged particle moving in a uniform magnetic field and losses 4% of its kinetic energy. The radius of curvature of its path changes by ______.
A short bar magnet has a magnetic moment of 0. 65 J T-1, then the magnitude and direction of the magnetic field produced by the magnet at a distance 8 cm from the centre of magnet on the axis is ______.
Two horizontal thin long parallel wires, separated by a distance r carry current I each in the opposite directions. The net magnetic field at a point midway between them will be ______.
