Advertisements
Advertisements
प्रश्न
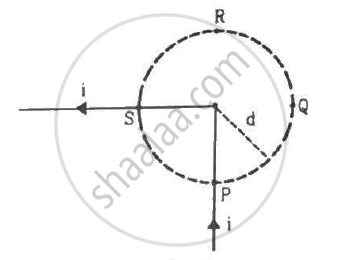
Figure shows a long wire bent at the middle to form a right angle. Show that the magnitudes of the magnetic fields at the point P, Q, R and S are equal and find this magnitude.
उत्तर
As shown in the figure, points P, Q, R and S lie on a circle of radius d.
Let the wires be named W1 and W2.
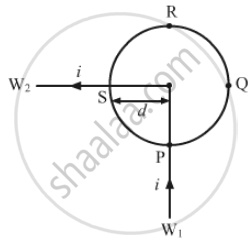
Now,
At point P, the magnetic field due to wire W1 is given by
B1 = 0
At point P, the magnetic field due to wire W2 is given by
\[B_2 = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in outward direction)
\[\Rightarrow B_{net} = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in outward direction)
At point Q, the magnetic field due to wire W1 is given by
\[B_1 = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in inward direction)
At point Q, the magnetic field due to wire W2 is given by
B2 = 0
\[\Rightarrow B_{net} = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in inward direction)
At point R, the magnetic field due to wire W1 is given by
B1 = 0
At point R, the magnetic field due to wire W2 is given by
\[\Rightarrow B_{net} = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in inward direction)
\[\Rightarrow B_{net} = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in inward direction)
At point S, the magnetic field due to wire W1 is given by
\[B_1 = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in outward direction)
At point S, the magnetic field due to wire W2 is given by
B2 = 0
\[\Rightarrow B_{net} = \frac{\mu_0 i}{4\pi d}\] (Perpendicular to the plane in outward direction)
Hence, the magnitude of the magnetic field at points P, Q, R and S is \[\frac{\mu_0 i}{4\pi d}\] .
APPEARS IN
संबंधित प्रश्न
Two concentric circular coils X and Y of radii 16 cm and 10 cm, respectively, lie in the same vertical plane containing the north to south direction. Coil X has 20 turns and carries a current of 16 A; coil Y has 25 turns and carries a current of 18 A. The sense of the current in X is anticlockwise, and clockwise in Y, for an observer looking at the coils facing west. Give the magnitude and direction of the net magnetic field due to the coils at their centre.
Two identical circular coils, P and Q each of radius R, carrying currents 1 A and √3A respectively, are placed concentrically and perpendicular to each other lying in the XY and YZ planes. Find the magnitude and direction of the net magnetic field at the centre of the coils.
At a place, the horizontal component of earth's magnetic field is B and angle of dip is 60°. What is the value of horizontal component of the earth's magnetic field at equator?
Use Biot-Savart's law to find the expression for the magnetic field due to a circular loop of radius 'r' carrying current 'I', at its centre ?
Derive the expression for the torque on a rectangular current carrying loop suspended in a uniform magnetic field.
A current-carrying, straight wire is kept along the axis of a circular loop carrying a current. This straight wire
Two circular coils of radii 5.0 cm and 10 cm carry equal currents of 2.0 A. The coils have 50 and 100 turns respectively and are placed in such a way that their planes as well as the centres coincide. Find the magnitude of the magnetic field B at the common centre of the coils if the currents in the coils are (a) in the same sense (b) in the opposite sense.
Find the magnetic field B due to a semicircular wire of radius 10.0 cm carrying a current of 5.0 A at its centre of curvature.
A circular loop of radius r carries a current i. How should a long, straight wire carrying a current 4i be placed in the plane of the circle so that the magnetic field at the centre becomes zero?
A circular coil of 200 turns has a radius of 10 cm and carries a current of 2.0 A. (a) Find the magnitude of the magnetic field \[\vec{B}\] at the centre of the coil. (b) At what distance from the centre along the axis of the coil will the field B drop to half its value at the centre?
A circular loop of radius 4.0 cm is placed in a horizontal plane and carries an electric current of 5.0 A in the clockwise direction as seen from above. Find the magnetic field (a) at a point 3.0 cm above the centre of the loop (b) at a point 3.0 cm below the centre of the loop.
Which of these equations is the correct expression for force on a charge in magnetic field?
A charged particle moving in a uniform magnetic field and losses 4% of its kinetic energy. The radius of curvature of its path changes by ______.
A small square loop of wire of side l is placed inside a large square loop of side L (L >> l). The loop is coplanar and their centers coincide. The mutual inductance of the system is proportional to is
Two horizontal thin long parallel wires, separated by a distance r carry current I each in the opposite directions. The net magnetic field at a point midway between them will be ______.
