Advertisements
Advertisements
प्रश्न
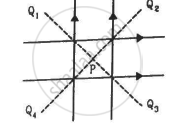
Four long, straight wires, each carrying a current of 5.0 A, are placed in a plane as shown in figure. The points of intersection form a square of side 5.0 cm.
(a) Find the magnetic field at the centre P of the square.
(b) Q1, Q2, Q3, and Q4, are points situated on the diagonals of the square and at a distance from P that is equal to the diagonal of the square. Find the magnetic fields at these points.
Advertisements
उत्तर
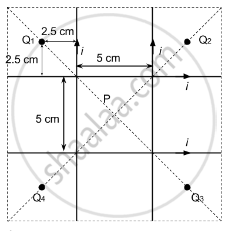
Given:
Let the horizontal wires placed at the bottom and top are denoted as W1 and W2 respectively.
Let the vertical wires placed at the right and left to point P are denoted as W3 and W4 respectively.
Magnitude of current, I = 5 A
(a) Consider point P.
Magnetic fields due to wires W1 and W2 are the same in magnitude, but they are opposite in direction.
Magnetic fields due to wires W3 and W4 are the same in magnitude, but they are opposite in direction.
Hence, the net magnetic field is zero.
Net magnetic field at P due to these four wires = 0
(b) Consider point Q1.
Due to wire W1, separation of point Q1 from the wire (d) is 7.5 cm.
So, the magnetic field due to current in the wire is given by
\[B_{W_1} = \frac{\mu_0 I}{2\pi d}\]
= 4 × 10−5 T (In upward direction)
Due to wire W2, separation of point Q1 from the wire (d) is 2.5 cm.
So, the magnetic field due to current in the wire is given by
\[B_{W_2} = \frac{4}{3} \times {10}^{- 5} T\] (In upward direction)
Due to wire W3, separation of point Q1 from the wire (d) is 7.5 cm.
So, the magnetic field due to current in the wire is given by
BW3 = 4 × 10−5 T (In upward direction)
Due to wire W4, separation of point Q1 from the wire (d) is 2.5 cm.
So, the magnetic field due to current in the wire is given by
\[B_{W_4} = \frac{4}{3} \times {10}^{- 5} T\] (In upward direction)
∴ Net magnetic field at point Q1
\[B_{Q_1} = \left( 4 + \frac{4}{3} + 4 + \frac{4}{3} \right) \times {10}^{- 5} \]
\[ = \frac{32}{3} \times {10}^{- 5} \]
\[ = 1 . 06 \times {10}^{- 4} T (\text{ In upward direction })\]
Magnetic field due to wire W1:
BW1 = 4 × 10−5 T (In upward direction)
Magnetic field due to wire W2:
∴ Net magnetic field at point Q2, \[B_{Q_2} = 0\]
APPEARS IN
संबंधित प्रश्न
Two infinitely long straight parallel wires, '1' and '2', carrying steady currents I1 and I2 in the same direction are separated by a distance d. Obtain the expression for the magnetic field `vecB`due to the wire '1' acting on wire '2'. Hence find out, with the help of a suitable diagram, the magnitude and direction of this force per unit length on wire '2' due to wire '1'. How does the nature of this force changes if the currents are in opposite direction? Use this expression to define the S.I. unit of current.
Using the concept of force between two infinitely long parallel current carrying conductors, define one ampere of current.
Two infinitely large plane thin parallel sheets having surface charge densities σ1 and σ2 (σ1 > σ2) are shown in the figure. Write the magnitudes and directions of the net fields in the regions marked II and III.
and ```vecE` and `vecB`denote electric and magnetic fields in a frame S and `vecE`→ and `vecB` in another frame S' moving with respect to S at a velocity `vecV` Two of the following equations are wrong. Identify them.
(a) `B_y^, = B_y + (vE_z)/c^2`
(b) `E_y^' = E_y - (vB_z)/(c^2)`
`(c) Ey = By + vE_z`
`(d) E_y = E_y + vB_z`
An electron is moving along the positive x-axis. You want to apply a magnetic field for a short time so that the electron may reverse its direction and move parallel to the negative x-axis. This can be done by applying the magnetic field along
(a) y-axis
(b) z-axis
(c) y-axis only
(d) z-axis only
A long, straight wire of radius R carries a current distributed uniformly over its cross section. T he magnitude of the magnetic field is
(a) maximum at the axis of the wire
(b) minimum at the axis of the wire
(c) maximum at the surface of the wire
(d) minimum at the surface of the wire.
A current of 10 A is established in a long wire along the positive z-axis. Find the magnetic field \[\vec{B}\] at the point (1 m, 0, 0).
A long, straight wire carrying a current of 1.0 A is placed horizontally in a uniform magnetic field B = 1.0 × 10−5 T pointing vertically upward figure. Find the magnitude of the resultant magnetic field at the points P and Q, both situated at a distance of 2.0 cm from the wire in the same horizontal plane.
The magnetic field existing in a region is given by `vecB = B_0(1 + x/1)veck` . A square loop of edge l and carrying a current i, is placed with its edges parallel to the x−y axes. Find the magnitude of the net magnetic force experienced by the loop.
Two long, straight wires, each carrying a current of 5 A, are placed along the x- and y-axis respectively. The currents point along the positive directions of the axes. Find the magnetic fields at the points (a) (1 m, 1 m), (b) (−1 m, 1 m), (c) (−1 m, −1 m) and (d) (1 m, −1 m).
A straight, how wire carries a current of 20 A. Another wire carrying equal current is placed parallel to it. If the force acting on a length of 10 cm of the second wire is 2.0 × 10−5 N, what is the separation between them?
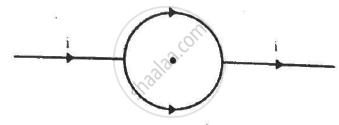
Define Ampere in terms of force between two current carrying conductors.
Equal currents are passing through two very long and straight parallel wires in the same direction. They will ______
Do magnetic forces obey Newton’s third law. Verify for two current elements dl1 = dlî located at the origin and dl2 = dlĵ located at (0, R, 0). Both carry current I.
Five long wires A, B, C, D and E, each carrying current I are arranged to form edges of a pentagonal prism as shown in figure. Each carries current out of the plane of paper.
- What will be magnetic induction at a point on the axis O? AxisE is at a distance R from each wire.
- What will be the field if current in one of the wires (say A) is switched off?
- What if current in one of the wire (say) A is reversed?
Two long straight parallel conductors carrying currents I1 and I2 are separated by a distance d. If the currents are flowing in the same direction, show how the magnetic field produced by one exerts an attractive force on the other. Obtain the expression for this force and hence define 1 ampere.
Beams of electrons and protons move parallel to each other in the same direction. They ______.
