Question

Construct ∆PQR such that m∠P = 80°, m∠Q = 70°, l(QR) = 5.7 cm.

Answer 1

In Δ PQR,

∠P + ∠Q + ∠R = 180^∘   ...(Angle sum property)

⇒ 80^∘ + 70^∘ + ∠R = 180^∘

⇒ 150^∘ + ∠R = 180^∘

⇒ ∠R = 180^∘ − 150^∘

= 30^∘ 

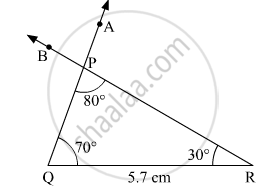
​Steps of construction:

(1) Draw seg QR of length 5.7 cm.

(2) Draw ray QA such that ∠RQA = 70°.

(3) Draw ray RB such that ∠QRB = 30°.

(4) Name the point of intersection of rays RB and QA as P.

Therefore, △PQR is the required triangle.