Advertisements
Advertisements
प्रश्न
Deduce an expression for equivalent capacitance C when three capacitors C1, C2 and C3 connected in parallel.
उत्तर
An expression for effective capacitance in the parallel grouping of capacitors :
Consider three capacitors of capacitance C1 , C2 and C3 are connected in parallel.
Let Q1, Q2 and Q3 be the charges deposited on the capacitors as shown in the figure.

Suppose a potential difference ‘V ’ is applied across the combination. Then, the potential difference between the plates of each capacitor is V but charges on each capacitor are different. Since different current flows through different branches, so the charges are given by
`Q_1 = C_1V.Q_2 = C_2V. Q_3 = C_3V` ....(i)
From the principle of conservation of charge
`Q = Q_1 + Q_2 + Q_3`
`Q = C_1V + C_2V + C_3V` [From equation (i)]
`∴ Q = V(C_1 + C_2 + C_3)`
If these capacitors are replaced by a single capacitor of capacity CP such that `Q = C_PV` then using equation (ii) we have,
`C_PV = V(C_1+C_2+C_3)`
`C_P = C_1+C_2+C_3`
APPEARS IN
संबंधित प्रश्न
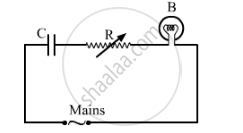
A capacitor 'C', a variable resistor 'R' and a bulb 'B' are connected in series to the ac mains in circuit as shown. The bulb glows with some brightness. How will the glow of the bulb change if (i) a dielectric slab is introduced between the plates of the capacitor, keeping resistance R to be the same; (ii) the resistance R is increased keeping the same capacitance?
Three capacitors each of capacitance 9 pF are connected in series.
- What is the total capacitance of the combination?
- What is the potential difference across each capacitor if the combination is connected to a 120 V supply?
Three capacitors of capacitances 2 pF, 3 pF and 4 pF are connected in parallel. Determine the charge on each capacitor if the combination is connected to a 100 V supply.
A circuit is set up by connecting inductance L = 100 mH, resistor R = 100 Ω and a capacitor of reactance 200 Ω in series. An alternating emf of \[150\sqrt{2}\] V, 500/π Hz is applies across this series combination. Calculate the power dissipated in the resistor.
Suppose a charge +Q1 is given to the positive plate and a charge −Q2 to the negative plate of a capacitor. What is the "charge on the capacitor"?
A parallel-plate capacitor is connected to a battery. A metal sheet of negligible thickness is placed between the plates. The sheet remains parallel to the plates of the capacitor.
Three capacitors of capacitance `C_1 = 3muf` , `C_2 = 6muf` , `C_3 = 10muf` , are connected to a 10V battery as shown in figure 3 below :
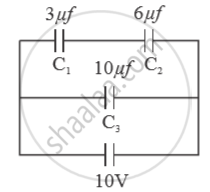
Calculate :
(a) Equivalent capacitance.
(b) Electrostatic potential energy stored in the system
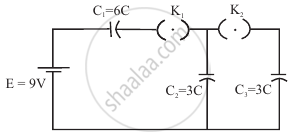
In the circuit shown in figure, initially K1 is closed and K2 is open. What are the charges on each capacitors.
Then K1 was opened and K2 was closed (order is important), What will be the charge on each capacitor now? [C = 1µF]