Advertisements
Advertisements
प्रश्न
Obtain an expression for an intensity of electric field at a point at the end of position, i.e., the axial position of an electric dipole.
x
उत्तर
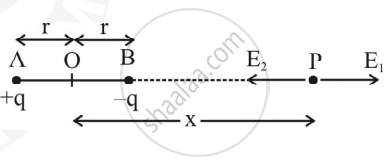
Consider an electric dipole consisting of charges –q and +q separated by a small distance 2r in free space.
Let P be a point on the axial line of the dipole at a distance x from the centre O of the dipole.
(i.e OP = x)
Electric field intensity at point P due to +q charge
`E_1 = 1/(4piepsilon_0).q/(AP^2)` (direction A to P)
`e_1 = 1/(4piepsilon_0).q/(x+r)^2` (direction A to P) ...(1)
Electric field intensity at point P due to –q charge
`E_2 = 1/(4piepsilon_0).q/(BP^2)` (direction P to B)
`E_2 = 1/(4piepsilon_0).q/(x-r)^2` (direction P to B) ...(2)
Since E2 > E1 and they act in opposite directions, resultant field intensity is given by :
E = E2 - E1 (direction P to B)
`= 1/(4piepsilon_0).q/(x-r)^2 - 1/(4piepsilon_0) .q/(x+r)^2`
`= 1/(4piepsilon_0).q[1/(x-r^2) - 1/(x+r)^2]`
`= 1/(4piepsilon_0).q[((x+r)^2 -(x-r)^2)/((x-r)^2 (x+r)^2)]`
`=1/4piepsilon_0.q[((x+r+x-r)(x+r-x+r))/(xx^2-r^2)^2]`
`= 1/4piepsilon_0.q [(2x.2r)/(x^2-r^2)^2]`
`E= 1/(4piepsilon_0). (2xp)/(x^2-r^2)^2 `[∵ p = 2r.q]
If the dipole is short (i.e., r << x) then r2 may be neglected as compared to x2
`E = 1/(4piepsilon_0) . (2p)/x^3`
The direction of resultant electric field E is along the dipole axis i.e., from –q charge to +q charge.
APPEARS IN
संबंधित प्रश्न
What is the work done by the field of a nucleus in a complete circular orbit of the electron? What if the orbit is elliptical?
The electrostatic potential energy of two-point charges, 1 µC each, placed 1 meter apart in the air is:
Two-point charges placed in a medium of dielectric constant 5 are at a distance r between them, experience an electrostatic force ‘F’. The electrostatic force between them in vacuum at the same distance r will be ____________.
If stretch in a spring of force constant k is tripled then the ratio of elastic potential energy in the two cases will be:
A work of 100 joule is performed in carrying a charge of 5 coulomb form infinity to a particular. point in an' electrostatic field. The potential of this point is:-
In bringing one electron towards another electrons, the electrostatic potential energy of the system will.
A point charge q0 is moving along a circular path of radius a, with a point charge Q at the centre of the circle. The kinetic energy of q0 is ______.
Three-point charges Q, q and -q are kept at the vertices of an equilateral triangle of side L as shown in the figure. What is
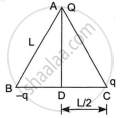
- the electrostatic potential energy of the arrangement? and
- the potential at point D?
