Advertisements
Advertisements
प्रश्न
Describe the vertical oscillations of a spring.
उत्तर
Vertical oscillations of a spring: Let us consider a massless spring with stiffness constant or force constant k attached to a ceiling as shown in the figure. Let the length of the spring before loading mass m be L. If the block of mass m is attached to the other end of the spring, then the spring elongates by a length l. Let F1 be the restoring force due to the stretching of spring. Due to mass m, the gravitational force acts vertically downward. We can draw a free-body diagram for this system as shown in the figure. When the system is under equilibrium,
F1 + mg = 0 …........(1)
But the spring elongates by small displacement l, therefore,
`"F"_1 ∝ "l" ⇒ "F"_1 = -"kl"` ...................(2)
Substituting equation (2) in equation (1), we get
−kl + mg = 0
mg = kl (or) `"m"/"k" = "l"/"g"` ..................(3)
Suppose we apply a very small external force on the mass such that the mass further displaces downward by a displacement y, then it will oscillate up and down. Now, the restoring force due to this stretching of spring (total extension of spring is y + l) is
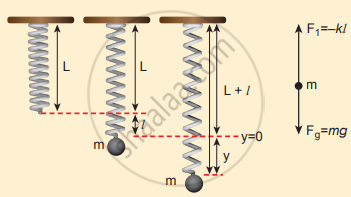
A massless spring with stiffness constant k
`"F"_2 ∝ ("y" + "l")`
F2 = −k(y + l) = −ky − kl .........(4)
Since, the mass moves up and down with acceleration `("d"^2"y")/"dt"^2`, by drawing the free body diagram for this case, we get
−ky − kl + mg = `"m"("d"^2"y")/"dt"^2` ......(5)
The net force acting on the mass due to this stretching is
F = F2 + mg
F = −ky − kl + mg .......(6)
The gravitational force opposes the restoring force. Substituting equation (3) in equation (6), we get
F = −ky − kl + kl = −ky
Applying Newton’s law, we get
`"m"("d"^2"y")/"dt"^2 = -"ky" ⇒ ("d"^2"y")/"dt"^2 = -"k"/"m""y"` .............(7)
The above equation is in the form of simple harmonic differential equation. Therefore, we get the time period as
T = `2π sqrt("m"/"k")` second .......(8)
The time period can be rewritten using equation (3)
T = `2π sqrt("m"/"k") = 2π sqrt("l"/"g")` ........(9)
The acceleration due to gravity g can be computed from the formula
g = `4π^2 ("l"/"T"^2) "ms"^(-2)` ...........(10)
APPEARS IN
संबंधित प्रश्न
Two bodies A and B whose masses are in the ratio 1 : 2 are suspended from two separate massless springs of force constants kA and kB respectively. If the two bodies oscillate vertically such that their maximum velocities are in the ratio 1 : 2, the ratio of the amplitude A to that of B is
A spring is connected to a mass m suspended from it and its time period for vertical oscillation is T. The spring is now cut into two equal halves and the same mass is suspended from one of the halves. The period of vertical oscillation is
The time period for small vertical oscillations of block of mass m when the masses of the pulleys are negligible and spring constant k1 and k2 is

A pendulum is hung in a very high building oscillates to and fro motion freely like a simple harmonic oscillator. If the acceleration of the bob is 16 ms−2 at a distance of 4 m from the mean position, then the time period is
Write down the equation of the time period for the linear harmonic oscillator.
Discuss the simple pendulum in detail.
Explain the horizontal oscillations of a spring.
Write short notes on the oscillations of the liquid column in the U-tube.
A piece of wood of mass m is floating erect in a liquid whose density is ρ. If it is slightly pressed down and released, then executes simple harmonic motion. Show that its time period of oscillation is T = `2π sqrt("m"/("Ag"ρ))`
Compute the time period for the following system if the block of mass m is slightly displaced vertically down from its equilibrium position and then released. Assume that the pulley is light and smooth, strings and springs are light.