Question

Draw graphs of displacement, velocity, and acceleration against phase angle, for a particle performing linear S.H.M. from

1. the mean position
2. the positive extreme position. 

Deduce your conclusions from the graph.

Answer 1

(a) Particle executing S.H.M., starting from mean position, towards positive:

Consider a particle performing S.H.M., with amplitude A and period T = 2π/ω starting from the mean position towards the positive extreme position where w is the angular frequency. Its displacement from the mean position (x), velocity (v), and acceleration (a) at any instant are

x = A sin  ωt = A sin ωt

v = `"dx"/"dt"` = Aω cos ωt

a = `"dv"/"dt"` = − Aω² sin ωt 

Using these expressions, the values of x, v, and a at the end of every quarter of a period, starting from t = 0, are tabulated below.

t	0	`"T"/4`	`"T"/2`	`"3T"/4`	T	`"5T"/4`
ωt	0	`π/2`	π	`"3π"/2`	2π	`(5pi)/2`
x	0	A	0	−A	0	A
v	Aω	0	−Aω	0	Aω	0
a	0	−Aω2	0	Aω2	0	-Aω2

Using the values in the table we can plot graphs of displacement, velocity, and acceleration with time.

(a)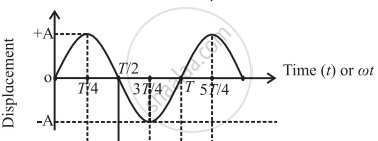
(b)
(c)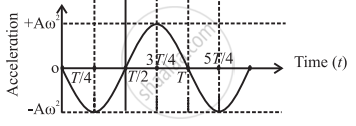

Graphs of displacement, velocity, and acceleration with time for a particle in SHM starting from the mean position

Conclusions:

• Displacement, velocity and acceleration of S.H.M. are periodic functions of time.
• Displacement time curve and acceleration time curves are sine curves and velocity time curve is a cosine curve.
• There is a phase difference of `π/2` radian between displacement and velocity.
• There is a phase difference of `π/2` radian between velocity and acceleration.
• There is a phase difference of π radian between displacement and acceleration.
• Shapes of all the curves get repeated after `2pi` radian or after a time T.

(b) Particle performing S.H.M., starting from the positive extreme position.

As the particle starts from the positive extreme position `phi = pi/2`

∴ Displacement, x = `A sin (omega"t" + pi/2) = A cos omega "t"`

∴ Velocity, v = `"dx"/"dt" = ("d"("A" cos omega"t"))/"dt" = - A omega sin (omega "t")`

∴ Acceleration,

a = `"dv"/"dt" = ("d"(- "A"omega sin (omega "t")))/"dt" = - Aomega^2 cos (omega "t")`

t	0	`"T"/4`	`"T"/2`	`"3T"/4`	T	`"5T"/4`
θ	`pi/2`	π	`"3π"/2`	`"2π"`	`(5π)/2`	`3pi`
x	A	0	-A	0	A	0
v	0	- Aω	0	Aω	0	- Aω
a	- Aω2	0	Aω2	0	- Aω2	0

[Phase θ = ωt + `phi`]

(a)
(b)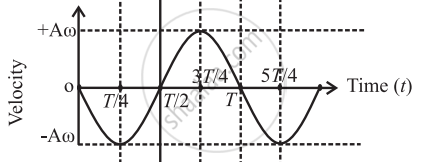
(c)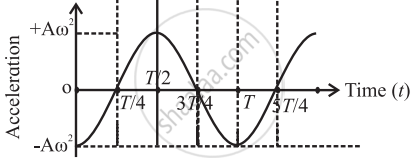

Conclusion -

1. The displacement, velocity and acceleration of a particle performing linear SHM are periodic (harmonic) functions of time. For a particle starting from an extreme position, the x-t and a-t graphs are cosine curves; the v-t graph is a sine curve.
2. There is a phase difference of `π/2` radians between x and v, and between v and a.
3. There is a phase difference of n radians between x and a.