Advertisements
Advertisements
प्रश्न
Draw the graph for each of the following equation: Also, find the coordinates of the points where the graph of the equation meets the coordinate axes:
`(3x + 14)/(2) = (y - 10)/(5)`
उत्तर
`(3x + 14)/(2) = (y - 10)/(5)`
⇒ 15x + 70 = 2y - 20
⇒ 15x - 2y = -90
⇒ 2y = 90 + 15x
⇒ y = `(90 + 15x)/(2)`
Corresponding values of x and y can be tabulated as follows :
| X | -5 | -2 | -1 |
| Y | 7.5 | 30 | 37.5 |
Plotting the points (-5, 7.5), (-2, 30) and (-1, 37.5),
we get the following graph :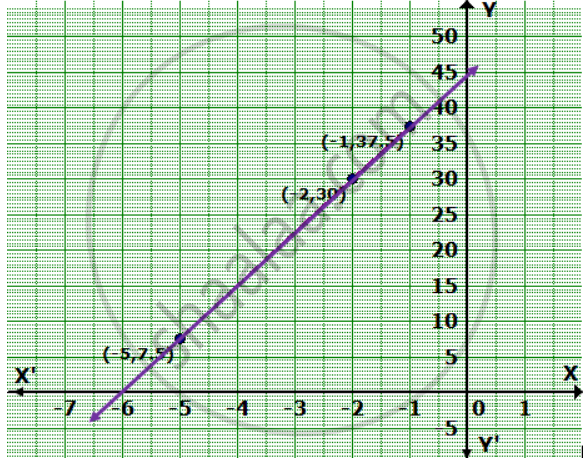
Thus, the graph of the equation meets the X-axis at (-6, 0) and Y-axis at (0, 45).
APPEARS IN
संबंधित प्रश्न
Plot the points (3, 5) and (− 1, 3) on a graph paper and verify that the straight line passing
through these points also passes through the point (1, 4).
Draw the graph of the equation given below. Also, find the coordinates of the point
where the graph cuts the coordinate axes : 2x + y = 6
Draw the graph of y = | x |.
Draw the graph for the equation, given below :
y = 7
Draw the graph for the equation, given below :
3x + 2y = 6
Draw the graph of the straight line given by the equation 4x - 3y + 36 = 0
Calculate the area of the triangle formed by the line drawn and the co-ordinate axes.
Draw the graph for the following
y = 2x
Draw the graph of the following equation:
y = 6
The graph of the linear equation y = x passes through the point ______.
The following observed values of x and y are thought to satisfy a linear equation. Write the linear equation:
| x | 6 | – 6 |
| y | –2 | 6 |
Draw the graph using the values of x, y as given in the above table. At what points the graph of the linear equation cuts the y-axis
