Advertisements
Advertisements
प्रश्न
Figure shows a square loop ABCD with edge-length a. The resistance of the wire ABC is r and that of ADC is 2r. Find the magnetic field B at the centre of the loop assuming uniform wires.
उत्तर
Let the currents in wires ABC and ADC be i1 and i2, respectively.
The resistances in wires ABC and ADC are r and 2r, respectively.
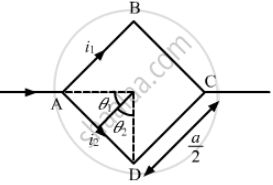
\[\therefore \frac{i_1}{i_2} = \frac{2}{1}\]
\[ \Rightarrow i_1 - 2 i_2 = 0 . . . \left( 1 \right)\]
And,
Now,
The magnetic field due to current in wire AD is given by
\[ \Rightarrow B = \frac{\mu_0 \frac{i}{3}}{4\pi\frac{a}{2}}(\sin45 + \sin45)\]
\[B' = 2B = \frac{\mu_0}{4\pi}\frac{i}{3}\frac{a}{a^2} \times 4 \times \sqrt{2}\]
\[ = \frac{\sqrt{2} \mu_0 i}{3\pi a}\]
(Perpendicular to the plane in outward direction)
The magnetic field at centre due to wire ABC is given by
\[B'' = \frac{\mu}{4\pi}\frac{2i}{3}\frac{a}{a^2} \times 4 \times \sqrt{2}\]
\[ = \frac{2\sqrt{2} \mu_0 i}{3\pi a}\]
(Perpendicular to the plane in inward direction)
(Perpendicular to the plane in inward direction)
APPEARS IN
संबंधित प्रश्न
Use Biot-Savart law to derive the expression for the magnetic field on the axis of a current carrying circular loop of radius R.
Draw the magnetic field lines due to a circular wire carrying current I.
At a place, the horizontal component of earth's magnetic field is B and angle of dip is 60°. What is the value of horizontal component of the earth's magnetic field at equator?
Using Biot-Savart law, deduce the expression for the magnetic field at a point (x) on the axis of a circular current carrying loop of radius R. How is the direction of the magnetic field determined at this point?
Use Biot-Savart's law to find the expression for the magnetic field due to a circular loop of radius 'r' carrying current 'I', at its centre ?
Derive the expression for the torque on a rectangular current carrying loop suspended in a uniform magnetic field.
A current-carrying, straight wire is kept along the axis of a circular loop carrying a current. This straight wire
Consider the situation shown in figure. The straight wire is fixed but the loop can move under magnetic force. The loop will

A steady electric current is flowing through a cylindrical conductor.
(a) The electric field at the axis of the conductor is zero.
(b) The magnetic field at the axis of the conductor is zero.
(c) The electric field in the vicinity of the conductor is zero.
(d) The magnetic field in the vicinity of the conductor is zero.
A circular loop of radius R carries a current I. Another circular loop of radius r(<<R) carries a current i and is placed at the centre of the larger loop. The planes of the two circles are at right angle to each other. Find the torque acting on the smaller loop.
Find the magnetic field B due to a semicircular wire of radius 10.0 cm carrying a current of 5.0 A at its centre of curvature.
A circular loop of radius r carries a current i. How should a long, straight wire carrying a current 4i be placed in the plane of the circle so that the magnetic field at the centre becomes zero?
A circular loop of radius 4.0 cm is placed in a horizontal plane and carries an electric current of 5.0 A in the clockwise direction as seen from above. Find the magnetic field (a) at a point 3.0 cm above the centre of the loop (b) at a point 3.0 cm below the centre of the loop.
Which of these equations is the correct expression for force on a charge in magnetic field?
The magnitude of the magnetic field due to a circular coil of radius R carrying a current I at an axial distance x from the centre is ______.
If we double the radius of a coil keeping the current through it unchanged, then the magnetic field at any point at a large distance from the centre becomes approximately.
A charged particle moving in a uniform magnetic field and losses 4% of its kinetic energy. The radius of curvature of its path changes by ______.
A short bar magnet has a magnetic moment of 0. 65 J T-1, then the magnitude and direction of the magnetic field produced by the magnet at a distance 8 cm from the centre of magnet on the axis is ______.
If ar and at represent radial and tangential accelerations, the motion of the particle will be uniformly circular, if:
